Question

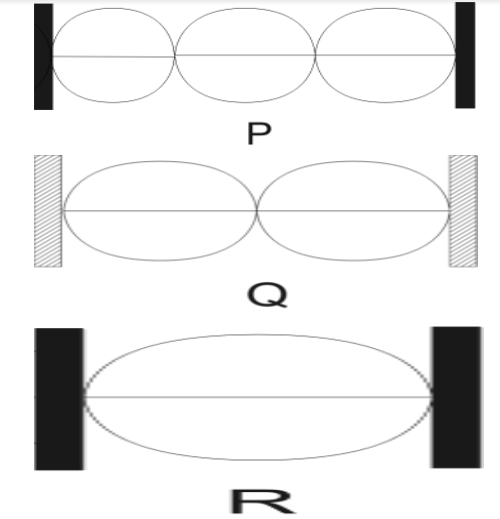
The diagram below shows the different modes of vibration P, Q and R of the same string of a given length,
(a) Which vibration will produce a louder sound and why?
(b) Which vibration will produce the sound of maximum shrillness (or pitch) and why?
(c) What is the ratio of the wavelength of vibrations P and R?

Answer 1

Hint:When sound waves travel through the air, then the air molecule vibrates, and hits the air molecule present next to it, which then also starts to vibrate. The air molecules do not "travel" with the wave. They start to vibrate. When the vibrations are fast (high frequency), you hear a high note. When vibrations are slower, you hear a lower note.

Complete Step by Step Solution:
(a):-
A sound wave which has a higher Amplitude will produce louder sound. It is the amplitude that defines the loudness of sound and the higher the amplitude, the louder the sound will be and vice versa.
From the above statement we can conclude that R will have a louder sound in all of the three options.

Hence, Option (R) is correct for question (a).

(b):-
Shrillness or Pitch depends on the Frequency of sound, The higher the frequency of sound the more pitch it will have.
So, we can say that P will produce the sound with maximum pitch because it has the highest frequency of all three.

Hence “P” is the correction answer for question (b).

(c):-
In a wave the distance between any given point and the same point in the next wave cycle is known as wavelength.
By the given figure we know that the wavelength of wave P is 3 times as that of wave R, because the wave P is repeating itself 3 times while wave R is only doing it once
Hence the ratio of wavelength of P to R is given as $\dfrac{{{\lambda }_{P}}}{{{\lambda }_{R}}}=\dfrac{3}{1}$

Hence the ratio of wavelength of P to wavelength of R will be $3:1$.

Note:
Frequency is the Repetition of the wavelength in a given unit time and to find the wavelength, we can use the formula,
$\lambda =\dfrac{v}{f}$
Where $\lambda $ is the wavelength, $v$ is the velocity and $f$is the frequency of the wave.