Question

The distance between any two tetrahedral voids formed on any body diagonal of a closest packed structure is $x\times a$ , where a is the edge length of the closest packed structure.
The value of x is:
[A] $\dfrac{1}{3}$
[B] $\dfrac{1}{2}$
[C] 2
[D] $\dfrac{3}{2}$

Answer 1

Hint: The term "closest packed structures" refers to the most tightly packed or space-efficient composition of crystal structures. Now try to recall that lattice that has the highest packing efficiency. Two tetrahedral voids are situated at each body diagonal of the lattice.

Complete Solution :
We know that the body diagonal of any polyhedron is a line connecting two vertices that do not lie on the same face.
Pictorially we can represent it as-

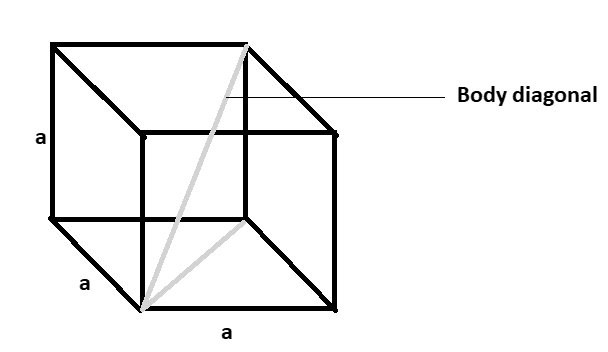
Where ‘a’ is the edge length which is equal for all the edges as it is a cube.
We know that tetrahedral voids are the voids formed by four spheres surrounding it from the four sides as in a regular tetrahedron.
Now, let us discuss the close packed structures-
- Face Centered Cubic (fcc) or Cubic Close Packed (ccp), these are two different names for the same lattice. We can think of this cell as being made by inserting another atom into each face of the simple cubic lattice - hence the "face-centered cubic" name.
- The number of tetrahedral and octahedral voids in CCP unit cells is 8 and 4 respectively. The number of particles in ccp is 4. Hence, the number of tetrahedral and octahedral voids is 8 and 4 respectively.
The void surrounded by four spheres sitting at the corners of a regular tetrahedron is called a tetrahedral void.

- We need to find the distance between two tetrahedral voids on the body diagonal -
If a is the edge length of the cube, then the length of body diagonal will be $a\sqrt{3}$. Half of this distance i.e. $\dfrac{a\sqrt{3}}{2}$ will be equal to the distance between the octahedral void (present at the center) and one corner.
- Tetrahedral voids are located on the diagonal of the cube at the one fourth distance from the corner i.e. $\dfrac{a\sqrt{3}}{4}$ .

- Now, we can write that the distance between the tetrahedral void and octahedral void is-$\dfrac{a\sqrt{3}}{2}-\dfrac{a\sqrt{3}}{4}=\dfrac{a\sqrt{3}}{4}$

So, we can say the distance between any two successive tetrahedral voids on body diagonal is $\dfrac{a\sqrt{3}}{2}$
Or, we can write it as $\dfrac{\sqrt{3}}{2}a$
So, the value of x is $\dfrac{\sqrt{3}}{2}$
So, the correct answer is “Option D”.

Note: We can also find the distance between any two successive tetrahedral voids. If we divide an FCC unit cell into 8 small cubes, then each small cube has 1 tetrahedral void at its own body centre.
Thus, there are 8 tetrahedral voids in total in one unit cell.
The nearest distance between two tetrahedral voids is $\dfrac{a}{2}$
In this case, the answer would be $\dfrac{1}{2}$.