Question

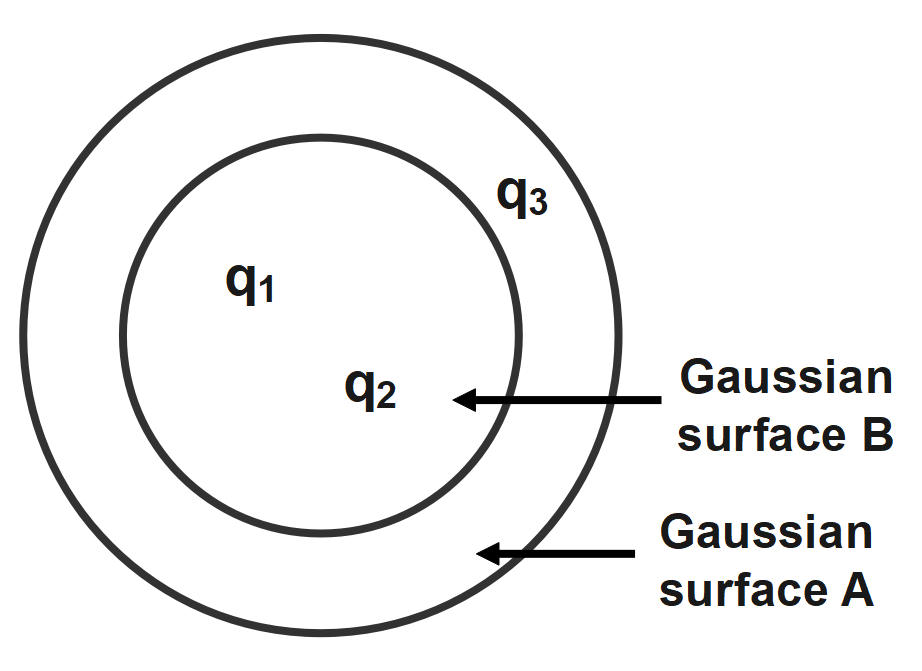
The electric flux for gaussian surface A that enclose the charged particles in free space is (given $ {{q}_{1}}=-14nC,\text{ }{{q}_{2}}=78.85nC,\text{ }{{q}_{3}}=-56nC $ ).

(A) $ {{10}^{3}}\left( N{{C}^{-1}}{{m}^{2}} \right) $
(B) $ {{10}^{3}}\left( C{{N}^{-1}}{{m}^{-2}} \right) $
(C) $ 6.32\times {{10}^{3}}\left( N{{C}^{-1}}{{m}^{2}} \right) $
(D) $ 6.32\times {{10}^{3}}\left( C{{N}^{-1}}m \right) $

Answer 1

Hint: We know that the electric flux passing through the point P will be dependent on the Gaussian surface and the charges it encloses, consistent with Gauss’s law. Hence, only the charges $ {{q}_{1}},\text{ }{{q}_{2}} $ and $ {{q}_{3}} $ will contribute to the electric flux in this region. The formula here for electric flux for gaussian surface used will be: $ {{\Phi }_{electric}}=\dfrac{Q}{{{\varepsilon }_{0}}} $ where $ {{\Phi }_{electric}} $ is electric flux; Q is the total charges enclosed per permittivity of free space; $ {{\varepsilon }_{0}} $ is permittivity of free space $ =8.85\times {{10}^{-12}}N{{C}^{2}}{{m}^{-2}} $ .

Complete step by step answer:
As we know, the electric flux due to the charges enclosed in a hypothetical Gaussian surface is always due to the charges enclosed within the surface. There is no contribution to the flux due to charge outside the Gaussian surface.
By calculating the flux by enclosing the charge in a hypothetical surface called Gaussian surface. The Gauss law states that the electric flux is due to some charges enclosed in a Gaussian surface. Thus, the total flux due to charges enclosed per permittivity of free space $ ({{\varepsilon }_{0}}) $ and given by $ Q={{q}_{1}}+{{q}_{2}}+{{q}_{3}}=\left( -14+78.85-56 \right)nC=8.85nC. $ Since we know for Charge unit that is nC stands nano Columb and nano is $ {{10}^{-9}}. $ $ \Rightarrow Q=8.85\times {{10}^{-9}}C. $
Now that we have total charges enclosed per $ {{\varepsilon }_{0}} $ we have to calculate electric flux for gaussian surface A that enclose the charged particles in free space is given by $ {{\Phi }_{electric}}=\dfrac{Q}{{{\varepsilon }_{0}}} $
On substitution the values in above equation we get;
 $ {{\Phi }_{electric}}=\dfrac{Q}{{{\varepsilon }_{0}}}=\dfrac{8.85\times {{10}^{-9}}\left( C \right)}{8.85\times {{10}^{-12}}\left( N{{C}^{2}}{{m}^{-2}} \right)} $ .
On further solving and taking denominator to numerator we get;
 $ {{\Phi }_{electric}}=\dfrac{{{10}^{-9}}\left( C \right)}{{{10}^{-12}}\left( N{{C}^{2}}{{m}^{-2}} \right)}={{10}^{-9}}\left( C \right)\times {{10}^{+12}}\left( N{{C}^{-2}}{{m}^{+2}} \right) $
On simplifying we get the value of electric flux:
 $ \Rightarrow {{\Phi }_{electric}}={{10}^{12-9}}\left( N{{C}^{-1}}{{m}^{2}} \right)={{10}^{3}}\left( N{{C}^{-1}}{{m}^{2}} \right). $
Therefore, correct answer is option A i.e. the electric flux for gaussian surface A that enclose the charged particles in free space is $ {{10}^{3}}\left( N{{C}^{-1}}{{m}^{2}} \right) $ .

Note:
Note that Coulomb's law can be derived from Gauss’s law and vice versa. It is one of Maxwell's four equations of electromagnetic interaction. It can be used to find the electric field due to discrete and continuous charge distributions.