
The electric potential due to an infinite sheet of positive charge density σ at a point located at a perpendicular distance Z from the sheet is (Assume
A.
B.
C.
D.
Answer
481.8k+ views
Hint: When we do any work against the conservative force then the work done by us will be stored in the form of potential energy in the system and potential energy increases. Along the direction of conservative force then potential energy of the system decreases.
Formula used:
Complete solution Step-by-Step:
Let us assume there is an object. We are displacing that object up very slowly which means at every instant we can assume its velocity will be zero. When we are moving an object upwards which means that we are displacing the object against the gravitational force. That literally means we are doing some work and according to conservation of energy that work will not go in vain. It will get converted in some form and that is nothing but in the form of potential energy.
If we clearly observe the above case, as the object is moving against gravity i.e as the work done by gravity is negative, the potential energy of the system is increasing.
Hence from the above relation we have the formula
Similarly electrostatic force is also conservative force.
Electric field due to the sheet is given as
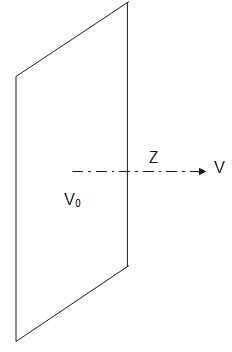
The above diagram depicts the sheet of positive charge and
From the above potential formula, we have
Hence, option D is correct.
Note:
Potential energy is valid only if conservative forces are present. Gravity, spring force and electrostatic force are the examples for conservative forces. In the question it doesn’t matter which coordinates they give, the electric field depends only upon the perpendicular distance from the sheet.
Formula used:
Complete solution Step-by-Step:
Let us assume there is an object. We are displacing that object up very slowly which means at every instant we can assume its velocity will be zero. When we are moving an object upwards which means that we are displacing the object against the gravitational force. That literally means we are doing some work and according to conservation of energy that work will not go in vain. It will get converted in some form and that is nothing but in the form of potential energy.
If we clearly observe the above case, as the object is moving against gravity i.e as the work done by gravity is negative, the potential energy of the system is increasing.
Hence from the above relation we have the formula
Similarly electrostatic force is also conservative force.
Electric field due to the sheet is given as

The above diagram depicts the sheet of positive charge and
From the above potential formula, we have
Hence, option D is correct.
Note:
Potential energy is valid only if conservative forces are present. Gravity, spring force and electrostatic force are the examples for conservative forces. In the question it doesn’t matter which coordinates they give, the electric field depends only upon the perpendicular distance from the sheet.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




