
The equation of a circle in parametric form is given by
(A)
(B)
(C)
(D)
Answer
507.9k+ views
Hint: Assume that A and B are the points of the tangency and the parametric angle of point A be
Complete step-by-step answer:
First of all, let us assume that A and B are the points of the tangency and the parametric angle of point A be
According to the question, it is given that the difference between the parametric angles of point A and point B is
It is also given that the parametric form of a point on the circle can be represented by
Since the parametric coordinate
On squaring equation (2) and equation (3), we get
On adding equation (4) and equation (5), we get
We know the identity,
From equation (6) and equation (7), we get
Now, we have got our equation of the circle,
We know the equation of the tangent at the point
The parametric angle of point and point B is
The coordinate of point A =
From equation (1), we have the value of
Now, the coordinate of point B =
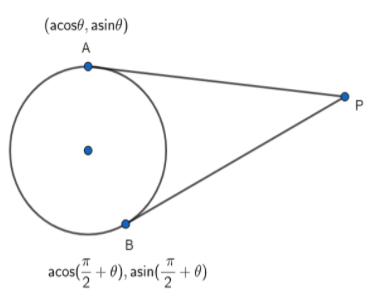
Using equation (8), we can get the equation of the tangent of the circle
Now, the equation of the tangent at point A i.e, AP
Similarly, using equation (8), we can get the equation of the tangent of the circle
Now, the equation of the tangent at point B i.e, BP
We know that,
From equation (12) and equation (13), we get
Since the tangents at points A and B are meeting at point P so, the point P must satisfy the equation of the tangent AP and BP.
Now, squaring equation (11) and equation (14), and adding then we get
From equation (7) and equation (15), we get
Therefore, the locus of the point of intersection is
Hence, option (B) is the correct one.
Note: In this question, one might take the parametric angle of point A as
We can see that the difference between the parametric angles of points A and B is not equal to
Complete step-by-step answer:
First of all, let us assume that A and B are the points of the tangency and the parametric angle of point A be
According to the question, it is given that the difference between the parametric angles of point A and point B is
It is also given that the parametric form of a point on the circle can be represented by
Since the parametric coordinate
On squaring equation (2) and equation (3), we get
On adding equation (4) and equation (5), we get
We know the identity,
From equation (6) and equation (7), we get
Now, we have got our equation of the circle,
We know the equation of the tangent at the point
The parametric angle of point and point B is
The coordinate of point A =
From equation (1), we have the value of
Now, the coordinate of point B =

Using equation (8), we can get the equation of the tangent of the circle
Now, the equation of the tangent at point A i.e, AP
Similarly, using equation (8), we can get the equation of the tangent of the circle
Now, the equation of the tangent at point B i.e, BP
We know that,
From equation (12) and equation (13), we get
Since the tangents at points A and B are meeting at point P so, the point P must satisfy the equation of the tangent AP and BP.
Now, squaring equation (11) and equation (14), and adding then we get
From equation (7) and equation (15), we get
Therefore, the locus of the point of intersection is
Hence, option (B) is the correct one.
Note: In this question, one might take the parametric angle of point A as
We can see that the difference between the parametric angles of points A and B is not equal to
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




