
The equation of the line of shortest distance between the lines
Answer
494.1k+ views
Hint: We needed to remember that the line of shortest distance between lines
Complete step-by-step solution:
From the given information, let us assume
Let
From the equation (1) we get
By using cross multiplication, we get
In the same way, from equation (1) we get
By using cross multiplication, we get
In the same way, from equation (3) we get
By using cross multiplication, we get
From equation (2), (3) and (4) let us assume a point on
Let
From the equation (5) we get
By using cross multiplication, we get
In the same way, from equation (5) we get
By using cross multiplication, we get
In the same way, from equation (5) we get
By using cross multiplication, we get
From equation (5), (6) and (7) let us assume a point on
We know that the equation of line passing through
Now we can find the equation of line passing through
We know that the line of shortest distance between lines
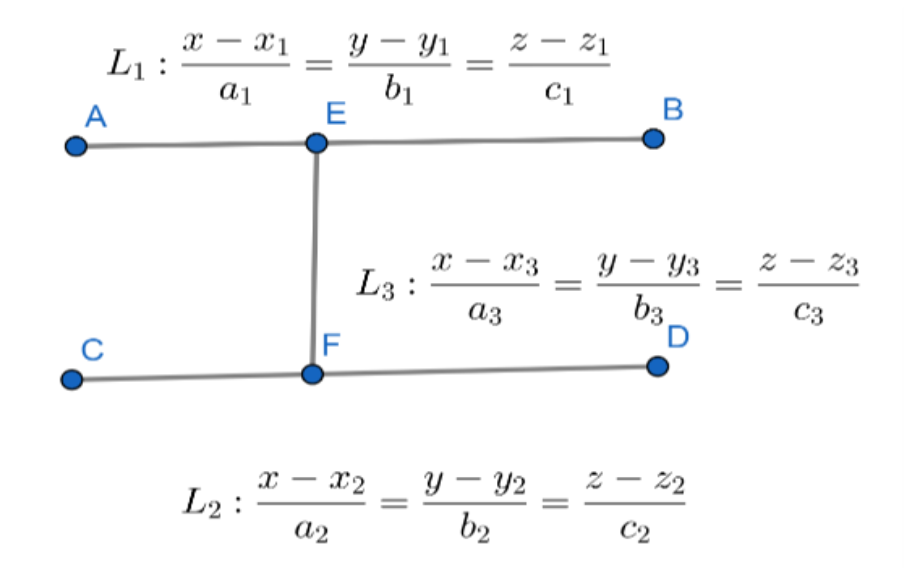
From the above condition, we get
We know that a line
So, Line
We get
In the similar manner, we know that line
We get
We need to find the values of
By solving equations (9) and (10) we will get the values of
We should multiply equation (9) by 7.
We should multiply (10) by 10.
Now we should subtract (11) and (12).
From equation (13) we get the value of
Now we will substitute the value of
From equation (14) we get the value of
Now we will substitute the both values of
Now we will multiply and divide the equation by (-3)
Hence, option (C) is correct.
Note: The formula to calculate the shortest distance between the lines between
Complete step-by-step solution:
From the given information, let us assume
Let
From the equation (1) we get
By using cross multiplication, we get
In the same way, from equation (1) we get
By using cross multiplication, we get
In the same way, from equation (3) we get
By using cross multiplication, we get
From equation (2), (3) and (4) let us assume a point on
Let
From the equation (5) we get
By using cross multiplication, we get
In the same way, from equation (5) we get
By using cross multiplication, we get
In the same way, from equation (5) we get
By using cross multiplication, we get
From equation (5), (6) and (7) let us assume a point on
We know that the equation of line passing through
Now we can find the equation of line passing through
We know that the line of shortest distance between lines

From the above condition, we get
We know that a line
So, Line
We get
In the similar manner, we know that line
We get
We need to find the values of
By solving equations (9) and (10) we will get the values of
We should multiply equation (9) by 7.
We should multiply (10) by 10.
Now we should subtract (11) and (12).
From equation (13) we get the value of
Now we will substitute the value of
From equation (14) we get the value of
Now we will substitute the both values of
Now we will multiply and divide the equation by (-3)
Hence, option (C) is correct.
Note: The formula to calculate the shortest distance between the lines between
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




