
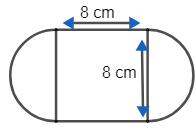
The figure above consists of a square and 2 semicircle, with dimensions as shown. Calculate its outside perimeter (in centimeters)
$\begin{align}
& \text{A}\text{. }8+8\pi \\
& \text{B}\text{. }16+8\pi \\
& \text{C}\text{. }16+16\pi \\
& \text{D}\text{. }32+8\pi \\
& \text{E}\text{. }32+16\pi \\
\end{align}$

Answer
501k+ views
Hint: To find the perimeter of the given figure, first we will find the outer surface of the semicircle which is also known as the circumference of a half circle. Then we will find the perimeter of the figure by adding all the sides of the figure.
Complete step by step solution:
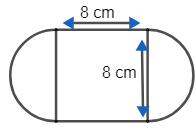
From the figure, we get to know that –
It consists of a square and 2 semicircles and the side of a square is equal to the diameter of the semicircle. i.e. $s=d=8$ …….. (1)
We know that –
$2\text{ Semicircles = Circle}$
Dividing both sides by 2, we get –
$\text{Semicircle=}\dfrac{1}{2}\text{Circle}$ …………………. (2)
We know that the perimeter of a circle is also known as circumference of a circle.
So the circumference of a $\dfrac{1}{2}$ circle $=\dfrac{1}{2}2\pi r$
Where, $r=\dfrac{d}{2}$ and $d=8$ (from equation 1)
So the circumference of a $\dfrac{1}{2}$ circle $=\dfrac{1}{2}\times 2\times \pi \times \dfrac{8}{2}$
By cancelling the common factor from numerator and denominator, we get –
$=4\pi $
Therefore, from equation (2) –
$\text{circumference of }\dfrac{1}{2}\text{ circle = circumference of semicircle =4}\pi $ …………………… (3)
Now, we will find the outside perimeter of the given figure.
The perimeter is the sum of all sides of the figure,
Let us consider, ‘s’ as the sides of squares and ‘c’ is the circumference of the semicircle.
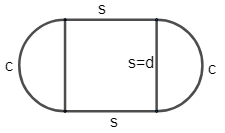
The perimeter of the given figure is $s+c+s+c$ .
By substituting the values from equation 1 and 3, we get that –
$\begin{align}
& 2s+2c=2\left( 8 \right)+2\left( 4\pi \right) \\
& =16+8\pi \\
\end{align}$
Therefore, the perimeter of the given figure is $16+8\pi $ .
Hence, the correct option is B.
Note: To solve this problem students must know that the length of a straight sided shapes outline is called perimeter, and the length of a circle’s outline is called its circumference. Here in this question there are 2 semi circles which is equal to a circle of same dimension
For example:
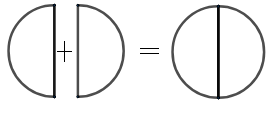
From the figure we can clearly see that the 2 semicircles of the same radius are equal to the circle of the same radius.
So, to find the perimeter of a circle we must need to find the circumference of semicircles.
Complete step by step solution:

From the figure, we get to know that –
It consists of a square and 2 semicircles and the side of a square is equal to the diameter of the semicircle. i.e. $s=d=8$ …….. (1)
We know that –
$2\text{ Semicircles = Circle}$
Dividing both sides by 2, we get –
$\text{Semicircle=}\dfrac{1}{2}\text{Circle}$ …………………. (2)
We know that the perimeter of a circle is also known as circumference of a circle.
So the circumference of a $\dfrac{1}{2}$ circle $=\dfrac{1}{2}2\pi r$
Where, $r=\dfrac{d}{2}$ and $d=8$ (from equation 1)
So the circumference of a $\dfrac{1}{2}$ circle $=\dfrac{1}{2}\times 2\times \pi \times \dfrac{8}{2}$
By cancelling the common factor from numerator and denominator, we get –
$=4\pi $
Therefore, from equation (2) –
$\text{circumference of }\dfrac{1}{2}\text{ circle = circumference of semicircle =4}\pi $ …………………… (3)
Now, we will find the outside perimeter of the given figure.
The perimeter is the sum of all sides of the figure,
Let us consider, ‘s’ as the sides of squares and ‘c’ is the circumference of the semicircle.

The perimeter of the given figure is $s+c+s+c$ .
By substituting the values from equation 1 and 3, we get that –
$\begin{align}
& 2s+2c=2\left( 8 \right)+2\left( 4\pi \right) \\
& =16+8\pi \\
\end{align}$
Therefore, the perimeter of the given figure is $16+8\pi $ .
Hence, the correct option is B.
Note: To solve this problem students must know that the length of a straight sided shapes outline is called perimeter, and the length of a circle’s outline is called its circumference. Here in this question there are 2 semi circles which is equal to a circle of same dimension
For example:
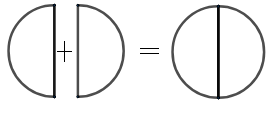
From the figure we can clearly see that the 2 semicircles of the same radius are equal to the circle of the same radius.
So, to find the perimeter of a circle we must need to find the circumference of semicircles.
Recently Updated Pages
Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Trending doubts
A number is chosen from 1 to 20 Find the probabili-class-10-maths-CBSE

Distinguish between the reserved forests and protected class 10 biology CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility

Difference between mass and weight class 10 physics CBSE




