
The figure shows a rectangle ABCD with a semicircle and a circle inscribed inside it as shown. What is the ratio of the area of the semi circle to that of the circle?
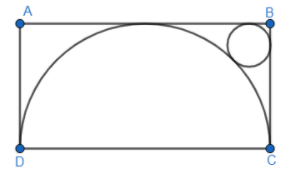

Answer
506.1k+ views
Hint: Start by drawing a figure with all necessary constructions and let the centre of DC and the semicircle be O and the point where the semi-circle is touching AB be P. Let the centre of the circle be C’, the point of intersection of the line CB with the circle be T. Also, let the radius of the semicircle be R and that of the circle be r. Now use the required construction and properties of the geometrical figures to find a relation between r and R. Finally find the ratio between
Complete step-by-step answer:
Let us start by drawing a figure with necessary points and constructions for better visualisation. We let the centre of DC and the semicircle be O and the point where the semi-circle is touching AB be P. Let the centre of the circle be C’, the point of intersection of the line C’B with the circle be T. Also, let the radius of the semicircle be R and that of the circle be r.
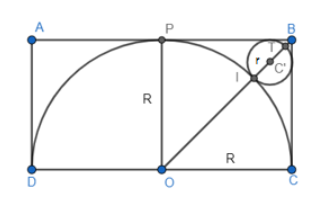
Now according to the given and assumed things:
OP=OC=PB=BC=R
Hence, POCB is a square. Therefore, we can say that OB is
Now looking at the diagram we can say:
Also, the perpendicular tangents of a circle lie on the director circle, i.e., the distance of the point of intersection of perpendicular tangents of a circle is at a distance of
Equation equation (i) and (ii), we get
Now we know that
Now, we know that the area of the circle is
Hence, the answer to the above question is
Note: Never put the value of
Complete step-by-step answer:
Let us start by drawing a figure with necessary points and constructions for better visualisation. We let the centre of DC and the semicircle be O and the point where the semi-circle is touching AB be P. Let the centre of the circle be C’, the point of intersection of the line C’B with the circle be T. Also, let the radius of the semicircle be R and that of the circle be r.

Now according to the given and assumed things:
OP=OC=PB=BC=R
Hence, POCB is a square. Therefore, we can say that OB is
Now looking at the diagram we can say:
Also, the perpendicular tangents of a circle lie on the director circle, i.e., the distance of the point of intersection of perpendicular tangents of a circle is at a distance of
Equation equation (i) and (ii), we get
Now we know that
Now, we know that the area of the circle is
Hence, the answer to the above question is
Note: Never put the value of
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




