Answer
388.2k+ views
Hint: This problem is based on Kirchhoff's current law. According to Kirchhoff’s current law the current entering the node is equal to the current leaving the node or the algebraic sum of the current meeting at the junction or node is equal to zero. To determine the magnitude or the amount of electrical current that is flowing around an electronic circuit or the electrical circuit, we need to utilize certain rules or the laws that make us write down these currents in the form of an equation.
Complete step by step answer:
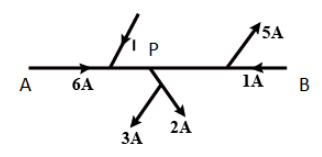
From the given circuit diagram, The current meeting at junction or node $P$ is
$I + 6 + 1$ ………. $\left( 1 \right)$
And , The current leaving at junction or node $P$ is
$5 + 3 + 2$………. $\left( 2 \right)$
Applying Kirchhoff’s current law to the given circuit, we can write the equation as
By using equation $\left( 1 \right)$ and equation $\left( 2 \right)$, current entering the junction or node is equal to the current leaving the node.
Therefore, It can be equated as
$I + 6 + 1 = 5 + 3 + 2$
On simplifying the above equation we get
$I + 7 = 10$
Therefore,
$I = 10 - 7 \\
\therefore I = 3\,A$
Hence, option B is correct.
Note: The Kirchhoff’s second law is also known as Kirchhoff’s voltage law which states that the algebraic sum of electromotive force $\left( {emf} \right)$ and the change in potential difference are equal to zero. Kirchhoff's first law or Kirchhoff’s current law obeys the law of conservation because it deals with conservation of the current entering and leaving a junction or node. This is because it has no other place to go as no charge is lost.
Complete step by step answer:

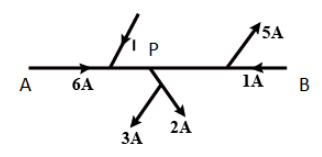
From the given circuit diagram, The current meeting at junction or node $P$ is
$I + 6 + 1$ ………. $\left( 1 \right)$
And , The current leaving at junction or node $P$ is
$5 + 3 + 2$………. $\left( 2 \right)$
Applying Kirchhoff’s current law to the given circuit, we can write the equation as
By using equation $\left( 1 \right)$ and equation $\left( 2 \right)$, current entering the junction or node is equal to the current leaving the node.
Therefore, It can be equated as
$I + 6 + 1 = 5 + 3 + 2$
On simplifying the above equation we get
$I + 7 = 10$
Therefore,
$I = 10 - 7 \\
\therefore I = 3\,A$
Hence, option B is correct.
Note: The Kirchhoff’s second law is also known as Kirchhoff’s voltage law which states that the algebraic sum of electromotive force $\left( {emf} \right)$ and the change in potential difference are equal to zero. Kirchhoff's first law or Kirchhoff’s current law obeys the law of conservation because it deals with conservation of the current entering and leaving a junction or node. This is because it has no other place to go as no charge is lost.
Recently Updated Pages
Identify the feminine gender noun from the given sentence class 10 english CBSE

Your club organized a blood donation camp in your city class 10 english CBSE

Choose the correct meaning of the idiomphrase from class 10 english CBSE

Identify the neuter gender noun from the given sentence class 10 english CBSE

Choose the word which best expresses the meaning of class 10 english CBSE

Choose the word which is closest to the opposite in class 10 english CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

Which are the Top 10 Largest Countries of the World?

Change the following sentences into negative and interrogative class 10 english CBSE

Difference between Prokaryotic cell and Eukaryotic class 11 biology CBSE

10 examples of friction in our daily life

How do you graph the function fx 4x class 9 maths CBSE

Give 10 examples for herbs , shrubs , climbers , creepers

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is pollution? How many types of pollution? Define it