
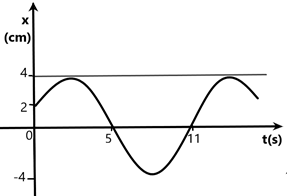
The figure shows the position time graph of an object in SHM. The correct equation representing this motion is –
\[\begin{align}
& \text{A) 2sin(}\dfrac{2\pi }{5}t+\dfrac{\pi }{6}) \\
& \text{B) 4sin(}\dfrac{\pi }{5}t+\dfrac{\pi }{6}) \\
& \text{C) 4sin(}\dfrac{2\pi }{6}t+\dfrac{\pi }{3}) \\
& \text{D) 4sin(}\dfrac{2\pi }{12}t+\dfrac{\pi }{6}) \\
\end{align}\]

Answer
460.8k+ views
Hint: We need to refer to the figure in which the position time graph variation is given. The equation of motion of a body undergoing a simple harmonic motion can be easily identified from its position-time graph which is given in this problem and so can be solved.
Complete answer:
We are given the position versus time graph of a body which is said to be undergoing simple harmonic motion. We know that the basic equation for a simple harmonic motion can be written as –
\[x=A\sin (\omega t+\phi )\]
Where, x is the displacement of the object at the time ‘t’, A is the maximum amplitude of displacement from the mean position, \[\omega \] is the angular frequency of the oscillation of the body and \[\phi \] is the initial phase difference if any.
We can see from the graph that we will get many quantities which can be used to find the equation of the simple harmonic motion. The maximum amplitude is the maximum value of displacement from the mean position, which is indicated on the graph as the maximum height from the time axis.
Here, the amplitude can be observed to be 4 cm in both directions (positive and negative x-axes).
Now, we can see that the point from which the harmonic motion starts on the x-axis is not at the origin. It is at a displacement of 2 cm from its mean position. With this we can find the initial phase difference as –
\[\begin{align}
& x=A\sin (\omega t+\phi ) \\
& \text{At }t=0,x=2cm \\
& \Rightarrow 2=4\sin (0+\phi ) \\
& \Rightarrow \dfrac{1}{2}=\sin \phi \\
& \therefore \phi =\dfrac{\pi }{6} \\
\end{align}\]
We get the phase difference as\[\dfrac{\pi }{6}\].
Now, we are given the one complete oscillation. The time taken for half of a complete oscillation is given as –
\[\begin{align}
& {{T}_{half}}=11-5\text{seconds} \\
& \Rightarrow {{T}_{half}}=6s \\
& \therefore T=12s \\
\end{align}\]
We can find the angular frequency from the time period as –
\[\begin{align}
& \omega =2\pi f \\
& \Rightarrow \omega =\dfrac{2\pi }{T} \\
& \therefore \omega =\dfrac{2\pi }{12}ra{{d}^{-1}} \\
\end{align}\]
Now, we have all the required unknowns to get the equation of the simple harmonic motion. So, we get the equation as –
\[\begin{align}
& x=A\sin (\omega t+\phi ) \\
& \therefore x=4\sin (\dfrac{2\pi }{12}t+\dfrac{\pi }{6}) \\
\end{align}\]
The correct answer is option D.
Note:
The equation of motion of a simple harmonic motion can be easily derived from the observations of basic measurable quantities such as the displacement from the mean position, its time period or the frequency and the initial displacement as we have done here.
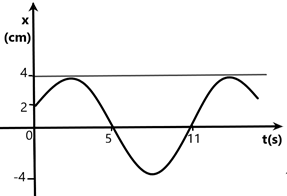
Complete answer:
We are given the position versus time graph of a body which is said to be undergoing simple harmonic motion. We know that the basic equation for a simple harmonic motion can be written as –
\[x=A\sin (\omega t+\phi )\]
Where, x is the displacement of the object at the time ‘t’, A is the maximum amplitude of displacement from the mean position, \[\omega \] is the angular frequency of the oscillation of the body and \[\phi \] is the initial phase difference if any.
We can see from the graph that we will get many quantities which can be used to find the equation of the simple harmonic motion. The maximum amplitude is the maximum value of displacement from the mean position, which is indicated on the graph as the maximum height from the time axis.
Here, the amplitude can be observed to be 4 cm in both directions (positive and negative x-axes).

Now, we can see that the point from which the harmonic motion starts on the x-axis is not at the origin. It is at a displacement of 2 cm from its mean position. With this we can find the initial phase difference as –
\[\begin{align}
& x=A\sin (\omega t+\phi ) \\
& \text{At }t=0,x=2cm \\
& \Rightarrow 2=4\sin (0+\phi ) \\
& \Rightarrow \dfrac{1}{2}=\sin \phi \\
& \therefore \phi =\dfrac{\pi }{6} \\
\end{align}\]
We get the phase difference as\[\dfrac{\pi }{6}\].
Now, we are given the one complete oscillation. The time taken for half of a complete oscillation is given as –
\[\begin{align}
& {{T}_{half}}=11-5\text{seconds} \\
& \Rightarrow {{T}_{half}}=6s \\
& \therefore T=12s \\
\end{align}\]
We can find the angular frequency from the time period as –
\[\begin{align}
& \omega =2\pi f \\
& \Rightarrow \omega =\dfrac{2\pi }{T} \\
& \therefore \omega =\dfrac{2\pi }{12}ra{{d}^{-1}} \\
\end{align}\]
Now, we have all the required unknowns to get the equation of the simple harmonic motion. So, we get the equation as –
\[\begin{align}
& x=A\sin (\omega t+\phi ) \\
& \therefore x=4\sin (\dfrac{2\pi }{12}t+\dfrac{\pi }{6}) \\
\end{align}\]
The correct answer is option D.
Note:
The equation of motion of a simple harmonic motion can be easily derived from the observations of basic measurable quantities such as the displacement from the mean position, its time period or the frequency and the initial displacement as we have done here.
Recently Updated Pages
What is the difference between Vapor pressure and Partial class 11 chemistry CBSE

Why is lanthanum in the Fblock class 11 chemistry CBSE

Select the correct order of stability of carbocation class 11 chemistry CBSE

Assertion Molar conductivity of a weak electrolyte class 11 chemistry CBSE

What is the maximum number of emission lines when the class 11 chemistry CBSE

What is the relation between amplitude of a wave and class 11 physics CBSE

Trending doubts
10 examples of friction in our daily life

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

State and prove Bernoullis theorem class 11 physics CBSE

What organs are located on the left side of your body class 11 biology CBSE

How many valence electrons does nitrogen have class 11 chemistry CBSE




