
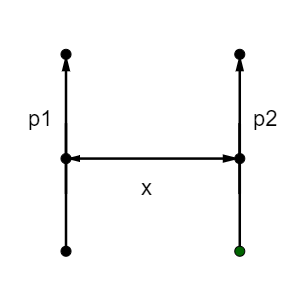
The force of interaction of two dipoles, if the two dipoles are parallel to each other and placed at distance x apart.
1. \[\dfrac{{3{p_1}{p_2}}}{{4\pi {\varepsilon _ \circ }{x^4}}}\]
2. \[\dfrac{{{p_1}{p_2}}}{{4\pi {\varepsilon _ \circ }{x^4}}}\]
3. \[\dfrac{{{p_1}{p_2}}}{{4\pi {\varepsilon _ \circ }{x^4}}}\]
4. \[\dfrac{{{p_1}{p_2}}}{{3\pi {\varepsilon _ \circ }{x^4}}}\]
Answer
142.8k+ views
Hint: First, we will need to find the electrostatic field of dipole \[{p_2}\] at \[{p_1}\] . Then we will find the potential energy of two dipoles. In the final step we will differentiate the potential energy to get the Force of interaction between two dipoles.
Complete step-by-step Solution
A dipole is separation of two opposite charges and it is quantified by electric dipole moment and is denoted by p.
As we know electric field of dipole along perpendicular bisector of the axis,
\[\overrightarrow E = - \dfrac{{\overrightarrow p }}{{4\pi {\varepsilon _ \circ }{r^3}}}\] , where r= distance
\[{\varepsilon _ \circ }\] = permittivity of free space
\[{E_{21}}\] is the field due to dipole \[{p_1}\] at dipole \[{p_2}\]
\[{E_{21}} = \dfrac{{{p_1}}}{{4\pi {\varepsilon _ \circ }{x^3}}}\]
Potential energy of dipole system
\[U = - \overrightarrow {{p_2}} .\overrightarrow {{E_{21}}} \]
\[U = - {p_2}\dfrac{{{p_1}}}{{4\pi {\varepsilon _ \circ }{x^3}}}\cos (\pi )\]
Angle between the dipole and electric field is 180 degrees.
\[U = \dfrac{{{p_1}{p_2}}}{{4\pi {\varepsilon _ \circ }{x^3}}}\]
Now, to find the force
\[F = - \dfrac{{dU}}{{dx}} = \dfrac{3}{{4\pi {\varepsilon _ \circ }}}\dfrac{{{p_1}{p_2}}}{{{x^4}}}\]
F is positive, so it is a repulsive force.
Option (1) \[\dfrac{{3{p_1}{p_2}}}{{4\pi {\varepsilon _ \circ }{x^4}}}\]
Additional Information
Electric field due to dipole at a general point
\[E = \dfrac{1}{{4\pi {\varepsilon _ \circ }}}\dfrac{p}{{{r^3}}}\sqrt {3{{\cos }^2}\theta + 1} \] , \[\theta \] =angle between the distance vector and dipole.
Potential due to dipole at a general point
\[V = \dfrac{{p\cos \theta }}{{4\pi {\varepsilon _ \circ }{r^2}}}\]
Note
1. You need to keep in mind the direction of the electric field and dipole.
2. While using the formula of potential energy of dipole, you need to find the angle between field and dipole otherwise you will get the wrong force direction.
3. While finding electric fields, Approximation is made that the length of the dipole is negligible as compared to the distance of the point from the dipole.
Complete step-by-step Solution
A dipole is separation of two opposite charges and it is quantified by electric dipole moment and is denoted by p.
As we know electric field of dipole along perpendicular bisector of the axis,
\[\overrightarrow E = - \dfrac{{\overrightarrow p }}{{4\pi {\varepsilon _ \circ }{r^3}}}\] , where r= distance
\[{\varepsilon _ \circ }\] = permittivity of free space
\[{E_{21}}\] is the field due to dipole \[{p_1}\] at dipole \[{p_2}\]
\[{E_{21}} = \dfrac{{{p_1}}}{{4\pi {\varepsilon _ \circ }{x^3}}}\]
Potential energy of dipole system
\[U = - \overrightarrow {{p_2}} .\overrightarrow {{E_{21}}} \]
\[U = - {p_2}\dfrac{{{p_1}}}{{4\pi {\varepsilon _ \circ }{x^3}}}\cos (\pi )\]
Angle between the dipole and electric field is 180 degrees.
\[U = \dfrac{{{p_1}{p_2}}}{{4\pi {\varepsilon _ \circ }{x^3}}}\]
Now, to find the force
\[F = - \dfrac{{dU}}{{dx}} = \dfrac{3}{{4\pi {\varepsilon _ \circ }}}\dfrac{{{p_1}{p_2}}}{{{x^4}}}\]
F is positive, so it is a repulsive force.
Option (1) \[\dfrac{{3{p_1}{p_2}}}{{4\pi {\varepsilon _ \circ }{x^4}}}\]
Additional Information
Electric field due to dipole at a general point
\[E = \dfrac{1}{{4\pi {\varepsilon _ \circ }}}\dfrac{p}{{{r^3}}}\sqrt {3{{\cos }^2}\theta + 1} \] , \[\theta \] =angle between the distance vector and dipole.
Potential due to dipole at a general point
\[V = \dfrac{{p\cos \theta }}{{4\pi {\varepsilon _ \circ }{r^2}}}\]
Note
1. You need to keep in mind the direction of the electric field and dipole.
2. While using the formula of potential energy of dipole, you need to find the angle between field and dipole otherwise you will get the wrong force direction.
3. While finding electric fields, Approximation is made that the length of the dipole is negligible as compared to the distance of the point from the dipole.
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Dual Nature of Radiation and Matter Class 12 Notes: CBSE Physics Chapter 11

Formula for number of images formed by two plane mirrors class 12 physics JEE_Main

Electrical Field of Charged Spherical Shell - JEE




