Question

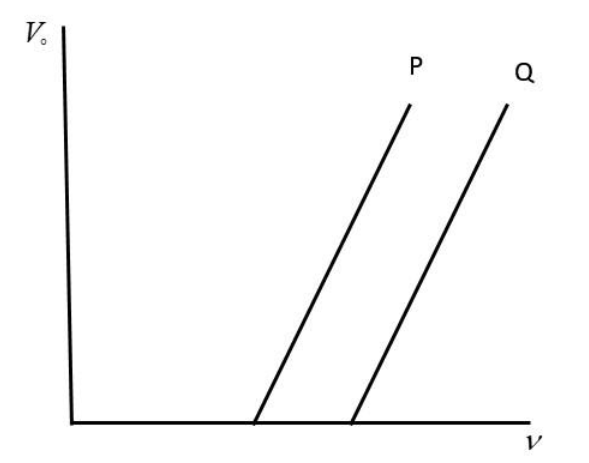
The graph between the stopping potential and frequency for two different metal plates P and Q are shown in the figure. Which of the metals has a greater threshold wavelength and work function?

Answer 1

Hint: Different material has different values of work function and stopping potential. It is the property of the material which defines how much energy is needed to eject an electron from the surface of a metal. For example, ejecting electrons out is easiest in metals such as tungsten.

Complete step-by-step solution:
Work function – It is defined as the minimum energy required to eject an electron from the surface of the material.
Threshold wavelength – It is defined as the maximum wavelength of the incident photon/ energy so that the electron can be ejected out of the surface.
Stopping potential – The minimum electric potential needed to stop the moving electron after getting ejected from the surface of the metal.
Now, coming to the graph, at the points where the lines cut the x-axis (i.e. frequency axis), the value of stopping potential is zero. That indicates that the electron is just ejected out (with zero velocity).
Now, according to the law of conservation of energy, the energy of the incident photon will go in first ejecting the electron and then in the kinetic energy of the electron. But the electrons which are just ejected from the surface, they won’t move and this means the energy incident is just sufficient to eject them. Hence it is a work function.
Now, we can see that for ‘p’, work function is less than that of ‘q’. And as threshold wavelength is inversely proportional to the work function, hence the threshold wavelength ‘p’ is greater than that of ‘q’.

Note: We can relate the above parameters. $W_\circ = h\nu = \dfrac{hc}{\lambda}$ where $W_\circ$ is the work function of the metal surface and $\nu\ and\ \lambda$ are threshold frequency and wavelength, respectively. It must be focused that we only want the graph for where the lines cut on the x-axis. At that point or at every point on the x-axis, the applied potential difference is zero hence a known value to us. Hence we can use this information.