
The graph of the equation of the form
A.
B.
C.
D.
Answer
497.4k+ views
Hint: We will first consider the graph of the general form of equation,
Complete step by step Answer:
As we will first consider the given equation of a line in the question, that is
We will first make the graph of the line
Thus, we get,
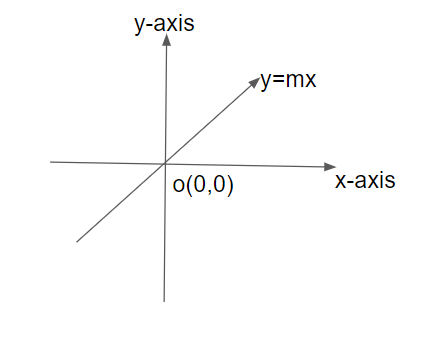
Now, the graph is of a straight line and from the graph we can see that the line
Thus, we can conclude that the equation
Hence, option D is correct.
Note: As the graph of the linear equation is always a straight line so we also have a linear equation whose graph is of a straight line. Also, every point on the straight line is the solution of the linear equation, and as we can observe that the line is passing through the origin. The general form of the equation with slope
Complete step by step Answer:
As we will first consider the given equation of a line in the question, that is
We will first make the graph of the line
Thus, we get,
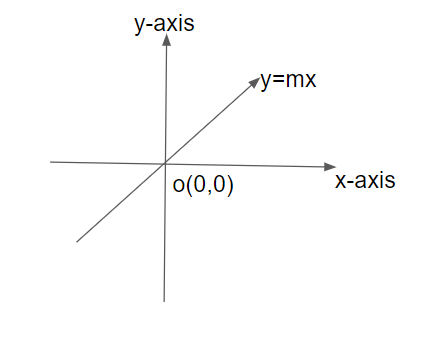
Now, the graph is of a straight line and from the graph we can see that the line
Thus, we can conclude that the equation
Hence, option D is correct.
Note: As the graph of the linear equation is always a straight line so we also have a linear equation whose graph is of a straight line. Also, every point on the straight line is the solution of the linear equation, and as we can observe that the line is passing through the origin. The general form of the equation with slope
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
The singular of lice is louse A Yes B No class 8 english CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

What led to the incident of Bloody Sunday in Russia class 8 social science CBSE




