Question

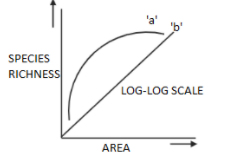
The graph shows the species-area relationship. Name the naturalist who studied two kinds of relationships shown in the graph. Write the observation made by him.

Answer 1

Hint: Species area relationship is also called species-area curve. Species area curve gives the relationship between the number of species found within that area and the part of habitat or the area of habitat. It was studied by a Prussian biologist.

Complete answer: Alexander Von Humboldt, a Prussian naturalist studied two kinds of relationships in the species-area curve. He observed an increase in a large number of species with the increase in the area but up to a certain limit. This relationship is represented using a rectangular parabola which describes the equation $S=c{{Z}^{2}}$. The $S$ in the equation stands for species richness, $A$ stands for the area, $c$ represents a constant term, and $z$ stands for the slope of the species-area relationship in log-log space. The straight line in the species-area relationship graph represents the logarithmic form of equation $S=c{{A}^{z}}$ which is \[\log \left( S \right)=\log \left( c \right)+z\log \left( A \right)\]. Various factors such as the relative balance between the immigration and extinction, predator-prey dynamics, rate and magnitude of disturbance on small vs. large areas, and clustering of individuals of the same species as a result of habitat heterogeneity or dispersal limitation are used to determine the slope and elevation of the species-area relationship.

Note: According to the census design used to construct species-area relationships, the species-area relationship differed. Species relationship curve is related to the species discovery curve but not identical to it. It is believed that the species-area relationship follows the second law of thermodynamics.