
The ionization energy of the hydrogen atom is 13.6 eV. A hydrogen atom in the ground state is excited by the electromagnetic radiation of energy 12.1 eV. How many spectral lines will be emitted by the hydrogen atom?
(A). One
(B). Two
(C). Three
(D). Four
Answer
518.1k+ views
Hint: Here we first calculate the difference between the ionization energy and the radiation energy. Then using the formula
Formula used:
Complete step-by-step solution -
Ionization energy denoted Eᵢ, is the minimum amount of energy required to remove the most loosely bound electron that is the valence electron, of an isolated neutral gaseous atom or molecule.
We know that the ionization energy of the hydrogen atom at the ground state is equal to -13.6 eV.
Now, this hydrogen atom is excited by electromagnetic energy equal to 12.1 eV.
So the energy difference is
that is the same as the energy of hydrogen atom in excited state
Now we know that the energy level of hydrogen is given by the formula
Here
Now equation (3) in equation (2) we will get
Therefore, Hydrogen is excited by radiation to the third energy state. Now the possible spectral lines that can be emitted are from
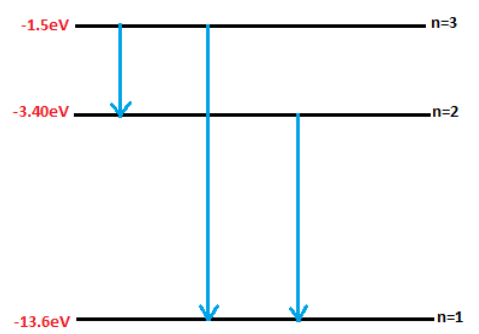
figure 1
Note: For these types of questions, we first find the energy difference ionization energy and radiation energy. Then recall the formula for the energy level of the atom which is
Formula used:
Complete step-by-step solution -
Ionization energy denoted Eᵢ, is the minimum amount of energy required to remove the most loosely bound electron that is the valence electron, of an isolated neutral gaseous atom or molecule.
We know that the ionization energy of the hydrogen atom at the ground state is equal to -13.6 eV.
Now, this hydrogen atom is excited by electromagnetic energy equal to 12.1 eV.
So the energy difference is
that is the same as the energy of hydrogen atom in excited state
Now we know that the energy level of hydrogen is given by the formula
Here
Now equation (3) in equation (2) we will get
Therefore, Hydrogen is excited by radiation to the third energy state. Now the possible spectral lines that can be emitted are from

figure 1
Note: For these types of questions, we first find the energy difference ionization energy and radiation energy. Then recall the formula for the energy level of the atom which is
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




