
The length of a focal chord of the parabola
A.
B.
C.
D.
Answer
501.3k+ views
Hint: We draw a rough figure for the situation where we have a parabola and a focal chord of length c. Using the property of vertically opposite angles we have the angle made by the chord with x-axis equal on both sides of the axis. With the help or right triangle formed by the shortest distance from the vertex to the chord we find cosecant of the angle. Substitute the value of cosecant of the angle in the formula for length of a chord.
* Focal chord is a chord that passes through the focus of the parabola
* In a right triangle having angle
*Length of focal chord of parabola
Complete step-by-step answer:
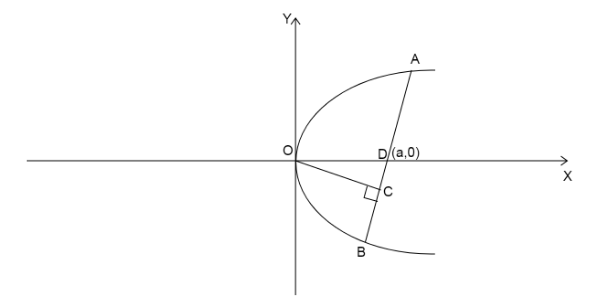
We are given a parabola
Let us assume that the chord cuts the X-axis at point D(a,0)
Then according to the question we are given the shortest distance from center to the chord is b.
Length of the focal chord is c.
The distance
Let us assume the focal chord makes an angle x with the X-axis.
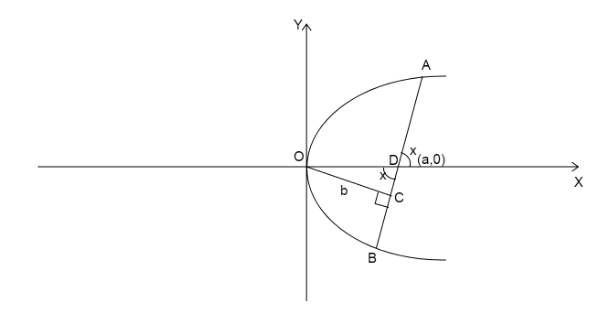
Since, we know vertically opposite angles are equal. Then,
Since, the shortest distance is the perpendicular distance, then
In right triangle OCD,
Substitute the value of OD as a and OC as b
Since we know
We know focal chord of a parabola
Therefore, we can write
Substitute the value of
Open the value on the bracket.
Cross multiply the value in denominator of RHS to LHS
Therefore, option D is correct.
Note: Students are likely to make the mistake of assuming the shortest distance as OD, which makes b equal to a. Keep in mind the shortest distance is the perpendicular distance and here the chord is not perpendicular to the x-axis so the perpendicular distance from vertex cannot be a.
* Focal chord is a chord that passes through the focus of the parabola
* In a right triangle having angle
*Length of focal chord of parabola
Complete step-by-step answer:

We are given a parabola
Let us assume that the chord cuts the X-axis at point D(a,0)
Then according to the question we are given the shortest distance from center to the chord is b.
Length of the focal chord is c.
The distance
Let us assume the focal chord makes an angle x with the X-axis.

Since, we know vertically opposite angles are equal. Then,
Since, the shortest distance is the perpendicular distance, then
In right triangle OCD,
Substitute the value of OD as a and OC as b
Since we know
We know focal chord of a parabola
Therefore, we can write
Substitute the value of
Open the value on the bracket.
Cross multiply the value in denominator of RHS to LHS
Therefore, option D is correct.
Note: Students are likely to make the mistake of assuming the shortest distance as OD, which makes b equal to a. Keep in mind the shortest distance is the perpendicular distance and here the chord is not perpendicular to the x-axis so the perpendicular distance from vertex cannot be a.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




