
The logical symbols shown here are logically equivalent to:
A) AND and OR gate
B) NOR and NAND gate
C) OR and AND gate
D) NAND and NOR gate


Answer
474.3k+ views
Hint: The given logic gates are OR and AND gates respectively but there’s a catch. The inputs being given to these logic gates have negated values. Since we are taking the sum and the product of the complements of terms A and B, we can approach this question using De Morgan’s Theorem.
Complete step by step solution:
De Morgan’s theorem is a powerful tool in digital design. The theorem explains that the complement of the product of all the terms is equal to the sum of the complement of each term.
Logically, we can depict this as
The inverse of the above statement is equally true. This means that the complement of the sum of all the terms is equal to the product of the complements of each term.
Logically, we can depict this as
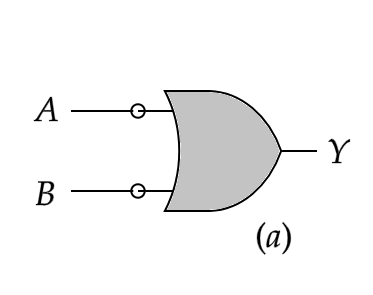
If we look at the two equations above from the right-hand side, the first equation is a representation of the logic gate marked
The truth table for the logic gate in figure (a) can be drawn as
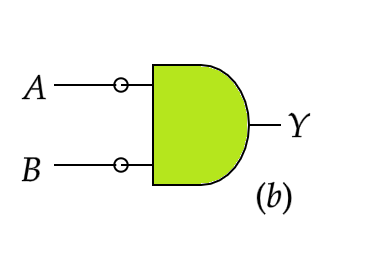
Similarly, we can draw the truth table for the logic gate in figure (b) as follows
Thus we can now say, both based on our argument, the truth tables and De Morgan’s theorem, that the two logic gates represent a NAND and a NOR gate respectively.
Hence the correct option is (D).
Note: We can check the validity of our argument and De Morgan’s theorem as well using a truth table. If we’ll make a truth table of the above two logic gates and compare them with the truth tables for a NAND and a NOR gate respectively. It should be noted that De Morgan’s theorem stands for any number of inputs.
Complete step by step solution:
De Morgan’s theorem is a powerful tool in digital design. The theorem explains that the complement of the product of all the terms is equal to the sum of the complement of each term.
Logically, we can depict this as
The inverse of the above statement is equally true. This means that the complement of the sum of all the terms is equal to the product of the complements of each term.
Logically, we can depict this as
If we look at the two equations above from the right-hand side, the first equation is a representation of the logic gate marked
The truth table for the logic gate in figure (a) can be drawn as
| 0 | 0 | 1 | 1 | 0 | 1 | 1 |
| 0 | 1 | 1 | 0 | 0 | 1 | 1 |
| 1 | 0 | 0 | 1 | 0 | 1 | 1 |
| 1 | 1 | 0 | 0 | 1 | 0 | 0 |
Similarly, we can draw the truth table for the logic gate in figure (b) as follows
| 0 | 0 | 1 | 1 | 0 | 1 | 1 |
| 0 | 1 | 1 | 0 | 1 | 0 | 0 |
| 1 | 0 | 0 | 1 | 1 | 0 | 0 |
| 1 | 1 | 0 | 0 | 1 | 0 | 0 |
Thus we can now say, both based on our argument, the truth tables and De Morgan’s theorem, that the two logic gates represent a NAND and a NOR gate respectively.
Hence the correct option is (D).
Note: We can check the validity of our argument and De Morgan’s theorem as well using a truth table. If we’ll make a truth table of the above two logic gates and compare them with the truth tables for a NAND and a NOR gate respectively. It should be noted that De Morgan’s theorem stands for any number of inputs.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




