Question

The magnifying power of a compound microscope is 20 and the distance between its two lenses is 30 cm when the final image is at the near point of the eye. If the focal length of the eyepiece is 6.25 cm the focal length of the objective is
a) 2.5cm
b) 3.5cm
c) 4.5cm
d) 5.0cm

Answer 1

Hint: A compound microscope basically consists of two lenses that is one is the objective and the and the other is the eyepiece. The objective has a very short focal length and is placed in front of the specimen. Whereas an eyepiece has a larger focal length than the objective. The idea of a compound microscope is that the image formed by the objective is placed within the focal length of the eye piece and hence we get a virtual and inverted image with respect to the object. The equation of magnifying power consists of the focal length of both eyepieces as well as the objective, the distance between the two lenses. Hence from the equation of the magnifying power we can calculate the focal length of the objective.

Complete step-by-step answer:

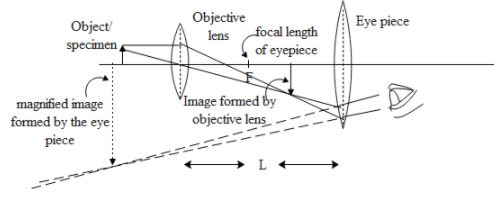
The above diagram represents a simple compound microscope whose magnifying power is given by,
$m=-\dfrac{L}{{{F}_{O}}}\left( 1+\dfrac{D}{{{F}_{E}}} \right)...(1)$ where L is the distance between the two lenses called as the tube length, D is the least distance for distinct vision i.e. for human eye it is 25 cm, ${{F}_{O}}$is the focal length of the objective and ${{F}_{E}}$is the focal length of the eye piece. Let us now find the focal length of the objective.
It is given in the question that, L=30 cm, D = -25 cm( minus sign indicates the distance is measured opposite to the incident light), m=20 and ${{F}_{E}}$= 6.25 cm. After substituting in equation 1 we get,
$\begin{align}
  & \text{m=-}\dfrac{\text{L}}{{{\text{F}}_{\text{O}}}}\left( \text{1+}\dfrac{\text{D}}{{{\text{F}}_{\text{E}}}} \right) \\
 & \text{20=-}\dfrac{\text{30cm}}{{{\text{F}}_{\text{O}}}}\left( \text{1-}\dfrac{\text{25cm}}{\text{6}\text{.25cm}} \right) \\
 & {{\text{F}}_{\text{O}}}\text{=}\dfrac{\text{30cm}}{\text{20}}\left( \text{1-4} \right) \\
 & {{\text{F}}_{\text{O}}}\text{=1}\text{.5cm}\left( \text{-3} \right)\text{=4}\text{.5cm} \\
\end{align}$
Hence the focal length of the objective is 4.5 cm.

So, the correct answer is “Option C”.

Note: In actual practice each of the objectives and the eyepiece consists of a combination of lenses. To eliminate chromatic aberration, an objective consists of two lenses in contact. To reduce spherical aberration and chromatic aberration an eye piece consists of two lenses separated by a certain distance.