Question

The number of lines of symmetry in regular pentagon is
A) 3
B) 4
C) 5
D) 6

Answer 1

Hint: Here we need to find the number of lines of symmetry in a regular polygon. Line of symmetry is defined as a line which divides the object into 2 equal parts. So here we will do the same i.e. we will try to find all possible lines across the regular polygon which will divide the regular polygon into two equal halves and the total possible numbers of such lines are our required answer.

Complete step by step solution:
Symmetry is defined as the quality of being made of exactly similar parts facing each other or around an axis.
The line of symmetry is defined as the axis or imaginary line that passes through the center of the shape or object and it divides into equal parts.
Regular pentagon is defined as the closed or plane figure that is made up of five equal sides and five equal angles.
If we fold the figure cut out at the center vertically, the halves will be congruent and the line of the fold is the line of the symmetry.
We can fold the regular pentagon 5 halves.
Now, we will draw the figure of a regular pentagon along with their line of symmetry.

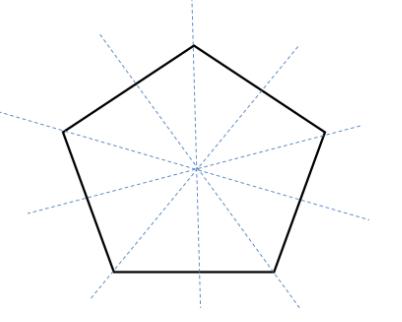
We can see from the figure that the number of lines of symmetry of regular is equal to 5.

Hence, the correct option is option (C).

Note:
Here we have obtained the number of lines of symmetry of a regular polygon. If we cut a paper in the shape of a polygon, then the line of fold which divides the polygon into two equal parts and that line will be the line of symmetry and number of such possible lines is 5 in case of regular polygon. In the same way, we can find the number of lines of symmetry of other shapes.