
The pedal equation of the circle
[a]
[b]
[c]
[d]
Answer
509.1k+ views
1 likes
Hint: Use the property that the tangent of a circle and the radius are perpendicular to each other at the point of contact. Use the property that angle in a semicircle is a right angle. Hence prove that the angles
Complete step-by-step answer:
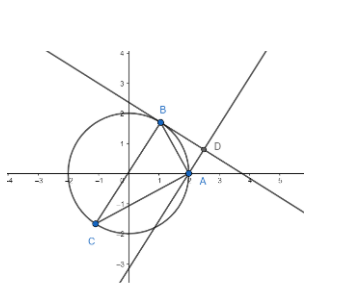
Pedal equation: Definition: Pedal equation of a curve C and given fixed point O is the relation between r and p, where r is the distance if point O to a point on the curve C and p is the perpendicular distance of O from the tangent line drawn at that point. The point O is called the pedal point and the values r and p are known as the pedal coordinates of the curve C w.r.t the pedal point.
Here we have, the pedal point is A (2,0), and B is any point on the locus. BD is the tangent at point B, and AD is the perpendicular from A on BD. C is the diametrically opposite point of B.
Hence, we have AD = p and AB = r.
Since BC is a diameter, we have
Let
Hence we have
Since BD is a tangent and BC is a diameter, we have
Hence, we have
In triangle ABD, we have
In triangle ABC, we have
From (i) and (ii), we get
Since for circle with equation
Put AD = p, AB = r and BC = 4, we get
which is the required pedal equation of the circle.
Hence option [b] is correct.
Note: In the pedal equation, we denote distance of any point on the locus from the pedal point by r and the distance of the pedal point from the tangent at the point on the locus by p. We then find the relation between p and r. This relation is called the pedal equation of the curve.
Alternative Solution:
Pedal equation of a circle with respect to any point on the circumference of the circle is given by
Here d = 4.
Hence the pedal equation is
Complete step-by-step answer:

Pedal equation: Definition: Pedal equation of a curve C and given fixed point O is the relation between r and p, where r is the distance if point O to a point on the curve C and p is the perpendicular distance of O from the tangent line drawn at that point. The point O is called the pedal point and the values r and p are known as the pedal coordinates of the curve C w.r.t the pedal point.
Here we have, the pedal point is A (2,0), and B is any point on the locus. BD is the tangent at point B, and AD is the perpendicular from A on BD. C is the diametrically opposite point of B.
Hence, we have AD = p and AB = r.
Since BC is a diameter, we have
Let
Hence we have
Since BD is a tangent and BC is a diameter, we have
Hence, we have
In triangle ABD, we have
In triangle ABC, we have
From (i) and (ii), we get
Since for circle with equation
Put AD = p, AB = r and BC = 4, we get
which is the required pedal equation of the circle.
Hence option [b] is correct.
Note: In the pedal equation, we denote distance of any point on the locus from the pedal point by r and the distance of the pedal point from the tangent at the point on the locus by p. We then find the relation between p and r. This relation is called the pedal equation of the curve.
Alternative Solution:
Pedal equation of a circle with respect to any point on the circumference of the circle is given by
Here d = 4.
Hence the pedal equation is
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




