Question

The perimeter of a scalene triangle and isosceles triangle and an equilateral triangle are equal, then which triangle has more area?
$\left( a \right)$ Equilateral
$\left( b \right)$ Isosceles
$\left( c \right)$ Scalene
$\left( d \right)$ Can’t say

Answer 1

Hint: In this particular question use the concept that Perimeter is nothing but the sum of all the sides of any 2 – dimensional shape, so the perimeter of the triangle is the sum of all the three sides so use these concepts to reach the solution of the question.

Complete step by step answer:

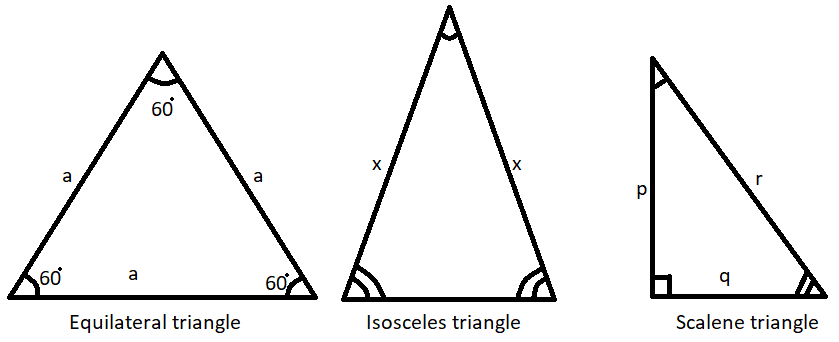
Equilateral triangle:
An equilateral triangle is a triangle in which all the sides of the triangle are equal and the measure of all the angles are also equal.
In a triangle the sum of all angles is 180 degrees.
So let that measure of one angle be x degrees.
So, ${x^o} + {x^o} + {x^o} = {180^o}$
$ \Rightarrow 3{x^o} = {180^o}$
$ \Rightarrow {x^o} = {60^o}$
So in an equilateral triangle the measure of all the angles are equal to 60 degrees, as shown in the figure.
Isosceles triangle:
An isosceles triangle is a triangle in which any of the lengths of two sides are equal and the length of the third side is different from the other two sides.
In an isosceles triangle opposite angles corresponding to similar sides are equal, and the opposite angle corresponding to different sides is different from the other two angles, as shown in the figure.
But the sum of all the angles remains 180 degrees.
Scalene triangle:
A scalene triangle is a triangle in which all the lengths of the sides are different w.r.t each other and the measures of all the angles are also different with each other.
Some right triangles can be a scalene triangle if the length of the legs of the right triangle is not the same, as shown in the figure.
Now it is given that the perimeter of a scalene triangle and isosceles triangle and an equilateral triangle are equal.
Perimeter is nothing but the sum of all the sides of any 2 – dimensional shape.
So the perimeter of the triangle is the sum of all the sides.
Now if all the sides of the triangle is given then the area of triangle is given as
\[\Delta = \sqrt {s\left( {s - a} \right)\left( {s - b} \right)\left( {s - c} \right)} \] Sq. units, where s is half of the perimeter and a, b, and c are the sides of the triangle.
As the perimeter of all the triangles are the same, the value of s is also same.
As in equilateral triangle all sides are same, in isosceles triangle two sides are same and in scalene triangle no sides are same, therefore
According to the property of triangles the maximum possible area covered by any triangle if the perimeter of all the triangles are equal is covered by an equilateral triangle.
So this is the required answer.

So, the correct answer is “Option a”.

Note: Whenever we face such types of questions the key concept we have to remember is that always recall the basic properties of a scalene triangle, isosceles triangle and an equilateral triangle which is all stated above and always recall that the maximum possible area covered by any triangle if the perimeter of all the triangles are equal is covered by an equilateral triangle. The area of the equilateral triangle can also be calculated by $\dfrac{{\sqrt 3 }}{4}{\left( {{\text{side}}} \right)^2}$.