
The potential difference across a 150mH inductor as a function of time is shown in figure. Assume that the initial value of the current in the inductor is zero. What is the current when


Answer
430.2k+ views
Hint: The voltage across the inductor is directly proportional to the rate of change of current through the inductor. When applied voltage is variable then integration logic is applied for solving such types of questions and we get current is directly proportional to the area of the Voltage-time graph.
Complete step-by-step solution:
The V - I equation of the inductor is given as:
-
Equation 1 can be written as,
As it is given in the question at time t = 0, current i = 0 and so let us assume at after time t current becomes i.
So on integrating above equation 2 from time 0 to t and current 0 to i , we get ,
According to the above equation current through the inductor is equal to the
Now we calculate current for
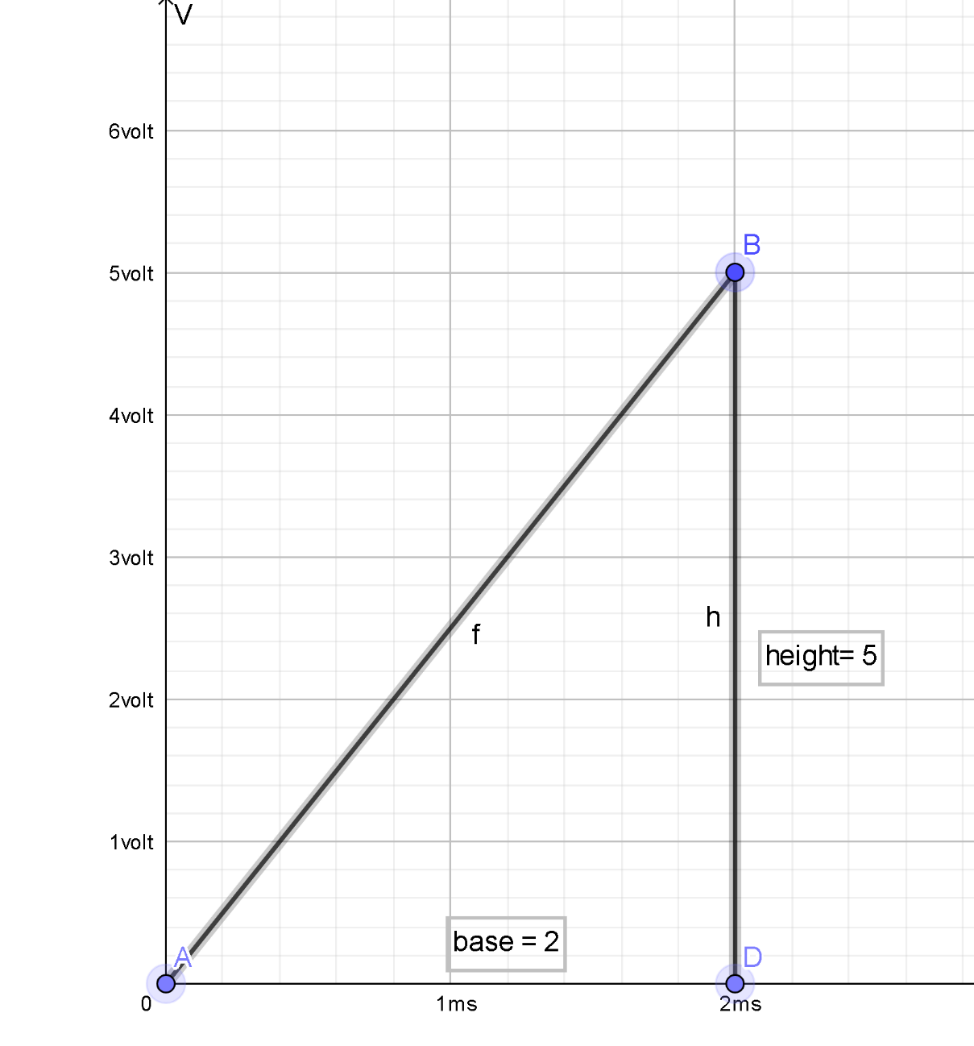
Now we have to find the current through the inductor at
On Solving we get ,
So, the value of current at
Now for

Similarly, to find the current through the inductor at t = 4.0ms we have to substitute the values below in equation 3.
So, the value of current at
So, we can conclude that current at
Note: Voltage in an inductor can only be calculated in the presence of changing current because changing current is having frequency. In the case of constant current passing through the inductor, the voltage is equal to zero and the inductor looks like a short circuit element.
Complete step-by-step solution:
The V - I equation of the inductor is given as:
-
Equation 1 can be written as,
As it is given in the question at time t = 0, current i = 0 and so let us assume at after time t current becomes i.
So on integrating above equation 2 from time 0 to t and current 0 to i , we get ,
According to the above equation current through the inductor is equal to the
Now we calculate current for

Now we have to find the current through the inductor at
On Solving we get ,
So, the value of current at
Now for

Similarly, to find the current through the inductor at t = 4.0ms we have to substitute the values below in equation 3.
So, the value of current at
So, we can conclude that current at
Note: Voltage in an inductor can only be calculated in the presence of changing current because changing current is having frequency. In the case of constant current passing through the inductor, the voltage is equal to zero and the inductor looks like a short circuit element.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




