Question

The radius of a circle is 5 m. Find the circumference of a circle whose area is 49 times the area of the given circle.

Answer 1

Hint: In this question it is given that the radius of a circle is 5 m. We have to find the circumference of a circle whose area is 49 times the area of the given circle. So before that let us draw the diagram,

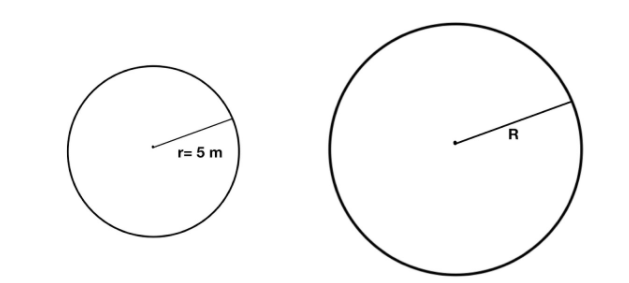
So to find the circumference of the bigger circle we need to find the radius of the bigger circle. So for this we need to know that, if the radius of a circle is r then the area of a circle is $$\pi r^{2}$$ and the circumference is $$2\pi r$$.

Complete step-by-step answer:
Here it is given the radius of the smaller circle is r=5 m.
Then the area of the circle,
 $$A_{1}=\pi r^{2}\ m^{2}=\pi \times 5^{2}\ m^{2}=25\pi \ m^{2}$$
Now let the radius of the bigger circle is R meter.
Then the area of the bigger circle,
$$A_{2}=\pi R^{2}$$
Since, it is given that the area of the bigger circle is 49 times the area of smaller circle,
Therefore we can write,
$$A_{2}=49A_{1}$$
$$\Rightarrow \pi R^{2}=49\times 25\pi$$
$$\Rightarrow R^{2}=\dfrac{49\times 25\times \pi }{\pi }$$
$$\Rightarrow R^{2}=49\times 25$$
$$\Rightarrow R=\sqrt{49\times 25}$$
$$\Rightarrow R=\sqrt{7\times 7\times 5\times 5}$$
$$\Rightarrow R=7\times 5$$ [ since, $$\sqrt{a\times a}= a$$]
$$\Rightarrow R=35$$
Therefore, the radius of the bigger circle is 35 m.
Then the circumference =$$2\pi R$$
  =$$2\times \dfrac{22}{7} \times 35$$ [
  =$$2\times \dfrac{22}{7} \times 5\times 7$$
  =$$2\times 22\times 5$$
   =$$220$$ m.

Hence the circumference of the circle is 220 m.

Note: While solving any circle related problems you need to know that the area and circumference of a circle depends upon its radius, so to find those quantities you first need to find the radius. Also apart from that you might be thinking why we used the term smaller and bigger circle, because here it was given that the area of the new circle is 49 times the previous circle, so it implies that the area of the new circle is more than the given circle, because of this reason we have used the term bigger and smaller.