
The ratio of packing density in fcc, bcc and cubic structure is respectively ______.
(A) 1 : 0.92 : 0.70
(B) 0.70 : 0.92 : 1
(C) 1 : 0.70 : 0.92
(D) 0.92 : 0.70 : 1
Answer
503.7k+ views
1 likes
Hint: Draw the diagram of a simple cubic, FCC and BCC unit cells. Each side of a simple cubic cell has length which equals two radii of two adjacent atoms. Volume of a sphere is equal to
Complete step by step answer:
For a simple cubic cell,
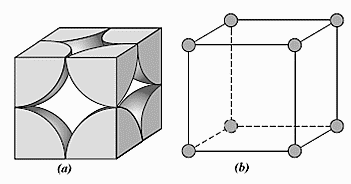
Length of each side of cubic cell, a=2r
Volume of one atom having radius (r) =
There are 8 atoms occupying the corners of the cube. Each corner contains only
Total volume of a unit cell
If an atom occupies the entire volume then, the volume occupied will be 100%. Now, the cubic cell contains only one atom and one atom can occupy only
Similarly, let us calculate for BCC unit cell,
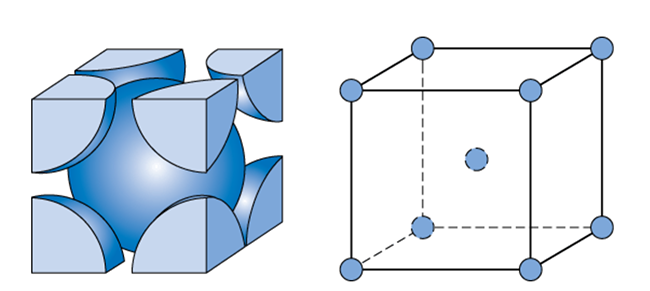
One atom is present in the centre of the unit cell and there are eight atoms present on the corners. Each corner contributes
Volume occupied by two atoms in the BCC unit cell
In a BCC unit cell, consider AD as the diagonal of the cube and AB as the diagonal of a side of a cube. Consider a right-angled triangle ABC, where (length of the side of the cube). Applying Pythagoras theorem to
Similarly, consider a right-angled triangle
But we know, the length of diagonal AD is 4r due to the presence of two radii of corner atoms and one diameter of central atom. So, equating the two values,
The total volume of cube
Therefore, packing efficiency of BCC unit cell is
Similarly, let’s calculate for FCC cells.
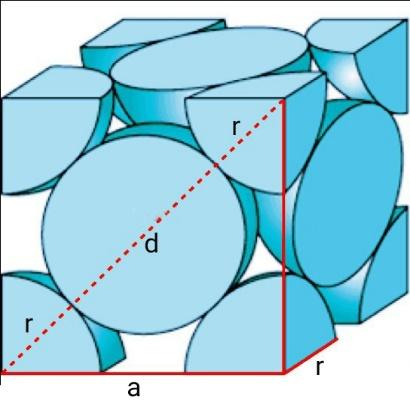
The FCC cell contains
Therefore, total atoms in a FCC cell
Consider a right-angled triangle
Therefore,
Volume of the FCC unit cell
Actual volume occupied by a cube of FCC
Hence, packing efficiency of FCC unit cell is
Now taking the ratio of all the three we get, FCC : BCC : SC=1 : 0.92 : 0.70
So, the correct answer is “Option A”.
Note: Systematically calculate the volume occupied by atoms in each of the unit cells. Empty space or void in each cell can be calculated as well by just subtracting packing efficiency by a factor of 100.
Complete step by step answer:
For a simple cubic cell,

Length of each side of cubic cell, a=2r
Volume of one atom having radius (r) =
There are 8 atoms occupying the corners of the cube. Each corner contains only
Total volume of a unit cell
If an atom occupies the entire volume then, the volume occupied will be 100%. Now, the cubic cell contains only one atom and one atom can occupy only
Similarly, let us calculate for BCC unit cell,

One atom is present in the centre of the unit cell and there are eight atoms present on the corners. Each corner contributes
Volume occupied by two atoms in the BCC unit cell
In a BCC unit cell, consider AD as the diagonal of the cube and AB as the diagonal of a side of a cube. Consider a right-angled triangle ABC, where (length of the side of the cube). Applying Pythagoras theorem to
Similarly, consider a right-angled triangle
But we know, the length of diagonal AD is 4r due to the presence of two radii of corner atoms and one diameter of central atom. So, equating the two values,
The total volume of cube
Therefore, packing efficiency of BCC unit cell is
Similarly, let’s calculate for FCC cells.

The FCC cell contains
Therefore, total atoms in a FCC cell
Consider a right-angled triangle
Therefore,
Volume of the FCC unit cell
Actual volume occupied by a cube of FCC
Hence, packing efficiency of FCC unit cell is
Now taking the ratio of all the three we get, FCC : BCC : SC=1 : 0.92 : 0.70
So, the correct answer is “Option A”.
Note: Systematically calculate the volume occupied by atoms in each of the unit cells. Empty space or void in each cell can be calculated as well by just subtracting packing efficiency by a factor of 100.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




