
The relation between RMS velocity, average velocity and most probable velocity is:
A: RMS velocity> average velocity > most probable velocity.
B: average velocity> RMS velocity> most probable velocity
C: RMS velocity= average velocity> most probable velocity
D: most probable velocity> average velocity> RMS velocity
Answer
437.7k+ views
Hint:
The kinetic molecular theory states that the gas molecules are continuously moving in random motion. During their motion, different molecules have different velocities and the molecules keep on colliding with each other. Velocity is used to describe the movement of gas molecules.
Complete step by step answer:
We need to derive the relation between the three types of velocities, that are RMS velocity, average velocity and most probable velocity. Before deriving the relation, we must know a little detail about them.
Root mean square velocity:
The relation between temperature and kinetic energy is
And the relation of kinetic energy is
On combining these two equations we get:
Or
Thus we can say that the root means square velocity as square root of average square velocity.
In the equation, R is gas constant, T is temperature and M is molar mass in kg/mol of molecule.
Average velocity:
Average velocity is the rate of change of position of an object. It can also be explained as total displacement in total time.
Most probable velocity:
The velocity which is possessed by the maximum fraction of gaseous molecules at a particular time is known as most probable velocity.
Its formula is:
If we compare these velocities, their ratio would be:
R,T and M are present in all the three so they will cancel out.
1.732: 1.596: 1.414
Therefore, the ratio of
It shows that
So RMS velocity> average velocity > most probable velocity.
Hence, the correct option Is (A).
Note:The distribution of magnitude of velocity of gas is shown with the help of maxwell-boltzmann curve.
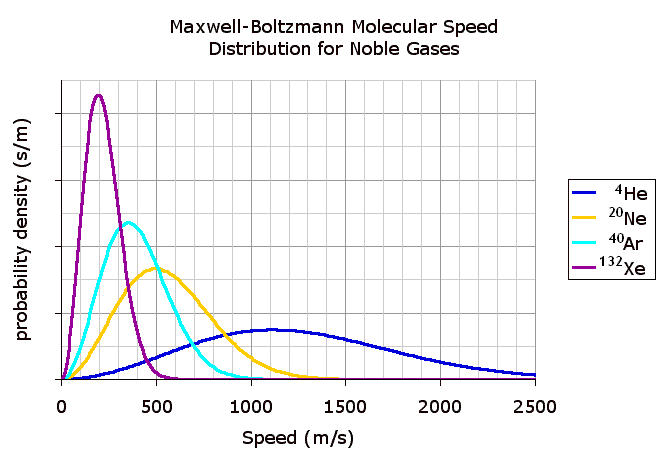
RMS velocity is used in place of average velocity because typical gas molecules move in all directions and have net zero velocity.
The kinetic molecular theory states that the gas molecules are continuously moving in random motion. During their motion, different molecules have different velocities and the molecules keep on colliding with each other. Velocity is used to describe the movement of gas molecules.
Complete step by step answer:
We need to derive the relation between the three types of velocities, that are RMS velocity, average velocity and most probable velocity. Before deriving the relation, we must know a little detail about them.
Root mean square velocity:
The relation between temperature and kinetic energy is
And the relation of kinetic energy is
On combining these two equations we get:
Or
Thus we can say that the root means square velocity as square root of average square velocity.
In the equation, R is gas constant, T is temperature and M is molar mass in kg/mol of molecule.
Average velocity:
Average velocity is the rate of change of position of an object. It can also be explained as total displacement in total time.
Most probable velocity:
The velocity which is possessed by the maximum fraction of gaseous molecules at a particular time is known as most probable velocity.
Its formula is:
If we compare these velocities, their ratio would be:
R,T and M are present in all the three so they will cancel out.
1.732: 1.596: 1.414
Therefore, the ratio of
It shows that
So RMS velocity> average velocity > most probable velocity.
Hence, the correct option Is (A).
Note:The distribution of magnitude of velocity of gas is shown with the help of maxwell-boltzmann curve.

RMS velocity is used in place of average velocity because typical gas molecules move in all directions and have net zero velocity.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




