Question

The resistive network shown below is connected to D.C. source of 16 V. The power consumed by the network is 4 watt. The value of R is:

$
  {\text{A}}{\text{. 8}}\Omega \\
  {\text{B}}{\text{. 6}}\Omega \\
  {\text{C}}{\text{. 1}}\Omega \\
  {\text{D}}{\text{. 16}}\Omega \\
 $

Answer 1

Hint: For the given circuit, first we need to find out the equivalent resistance for the given combination of resistances. Then by inserting the equivalent resistance in the formula for the power consumed in an electrical circuit, we can find out the values of R.

Formula Used
The power consumed by an electrical circuit is given as follows:
\[P = \dfrac{{{V^2}}}{R}\]


Complete step by step answer:
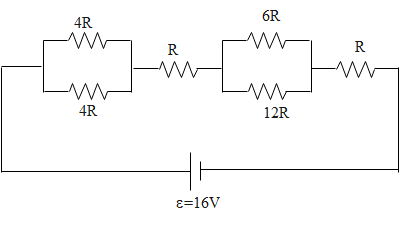
We are given a resistive network as shown in the diagram. Let us find out the equivalent resistance for the given combination of resistances.
The first two resistances are connected in parallel with each other. If R’ is their equivalent resistance then it can be obtained in the following way.
$R' = \dfrac{{4R \times 4R}}{{4R + 4R}} = \dfrac{{4R \times 4R}}{{8R}} = 2R$
Similarly, if R’’ is the equivalent resistance of the second set of resistance connected in parallel with each other then it can be obtained in the following way.
$R'' = \dfrac{{6R \times 12R}}{{6R + 12R}} = \dfrac{{6R \times 12R}}{{18R}} = 4R$
Now the above two equivalent resistances are connected in series with other resistance present in the circuit. Therefore, if R’’’ is the equivalent resistance of the complete circuit then it can be obtained in the following way.
$R''' = R' + R + R'' + R = 2R + R + 4R + R = 8R$
Now we are given the value of the voltage for the attached battery. Its value is given as
$\varepsilon = 16V$
The value of power is given to us which is
$P = 4W$
Now, the power consumed by this electrical circuit is given as follows:
$P = \dfrac{{{\varepsilon ^2}}}{{R'''}}$
Now inserting the known values, we obtain
$
  4 = \dfrac{{{{16}^2}}}{{8R}} \\
   \therefore R = \dfrac{{{{16}^2}}}{{8 \times 4}} = 8\Omega \\
 $
This is the required value of R. Hence, the required answer is option A.

Note:
It should be noted that when we take a parallel combination then the equivalent resistance is smaller than the individual resistances attached in parallel with each other. But for the resistances connected in series, the equivalent resistance adds up and is greater than the individual resistances attached in series with each other.