
The resultant magnetic moment of the following arrangement is:
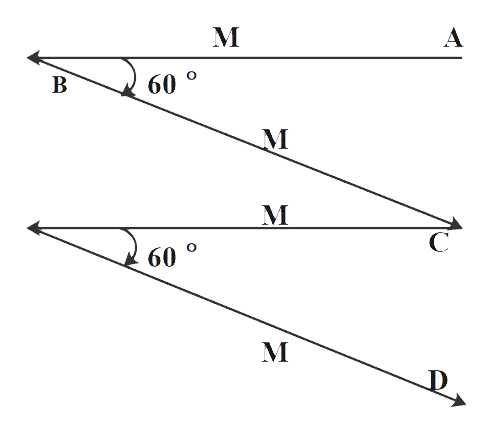
A. M
B. 2M
C. 3M
D. 4M

Answer
498.3k+ views
Hint: In this question we can see that there are two different sets of the magnetic moments. In each set the magnetic moments are parallel to each other i.e. A is parallel to C and B is parallel to D. So, we will add these as they have the same direction and same magnitude. Later, we will be using the formula of parallelogram law of vector addition.
Formula used:
Where,
The variable a and b represent the vectors and
Complete answer:
As we can see, the magnetic moments A and C are parallel to each other. So, their net magnetic moment will be given as 2M in the same direction. Similarly, the other two vectors B and D are also parallel to each other, so their net magnetic moment is also given as 2M. Therefore, we have two magnetic moments as shown in figure (a).
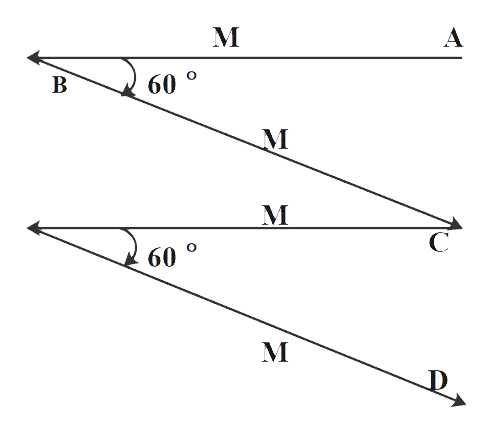
So, if we make these two magnetic vectors coplanar, we get vectors as shown in figure (b). The angle (
Now to find them we can use the parallelogram law of vector addition as the magnetic moment is a vector quantity.
We know the formula for parallelogram law of vector addition is,
We have,
Vector a =b = 2M and
So, after substituting these above values in our equation
We get,
On solving
We get,
So, the correct answer is “Option B”.
Additional Information:
The direction of the resultant vector is not asked but in case it is asked we can find the angle (
Note:
We can also solve this question by finding the resultant magnetic field between vector a and b. And then for c and d. We will then use the parallelogram law of vector addition and get the same result with different values. But, that would be confusing and time consuming.
Formula used:
Where,
The variable a and b represent the vectors and
Complete answer:
As we can see, the magnetic moments A and C are parallel to each other. So, their net magnetic moment will be given as 2M in the same direction. Similarly, the other two vectors B and D are also parallel to each other, so their net magnetic moment is also given as 2M. Therefore, we have two magnetic moments as shown in figure (a).

So, if we make these two magnetic vectors coplanar, we get vectors as shown in figure (b). The angle (
Now to find them we can use the parallelogram law of vector addition as the magnetic moment is a vector quantity.
We know the formula for parallelogram law of vector addition is,
We have,
Vector a =b = 2M and
So, after substituting these above values in our equation
We get,
On solving
We get,
So, the correct answer is “Option B”.
Additional Information:
The direction of the resultant vector is not asked but in case it is asked we can find the angle (
Note:
We can also solve this question by finding the resultant magnetic field between vector a and b. And then for c and d. We will then use the parallelogram law of vector addition and get the same result with different values. But, that would be confusing and time consuming.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




