
The speed of light in air and glass is
A.
B.
C.
D.
Answer
451.8k+ views
Hint: As a first step, you could make a ray diagram just to be clear about the situation. Then you could find the refractive index of glass from given values of speed of light in the two mediums. Then, you could use Snell's law to find the angle of refraction that took place in the glass and hence the answer.
Formula used:Snell’s law,
Complete step by step answer:
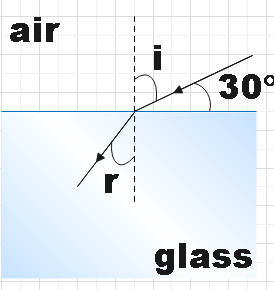
In the question, we are given the speed of light in two mediums which are air and glass.
The light is incident by making
We know that the refractive index of a medium is the speed of light with respect to the speed of light in air. So, the refractive index of glass would be,
Now from Snell’s law we have,
Substituting the values,
Therefore, we found the refracted angle to be,
So, the correct answer is “Option B”.
Note: One should always be careful while noting down the given angles. You shouldn’t forget that the incident angle or reflected angle, both are the angle made by the incident light with the normal and not the surface. In the question we are given the angle made with the surface and hence we have substituted accordingly.
Formula used:Snell’s law,
Complete step by step answer:

In the question, we are given the speed of light in two mediums which are air and glass.
The light is incident by making
We know that the refractive index of a medium is the speed of light with respect to the speed of light in air. So, the refractive index of glass would be,
Now from Snell’s law we have,
Substituting the values,
Therefore, we found the refracted angle to be,
So, the correct answer is “Option B”.
Note: One should always be careful while noting down the given angles. You shouldn’t forget that the incident angle or reflected angle, both are the angle made by the incident light with the normal and not the surface. In the question we are given the angle made with the surface and hence we have substituted accordingly.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
What is the difference between resemblance and sem class 12 social science CBSE

Draw ray diagrams each showing i myopic eye and ii class 12 physics CBSE

Why do the transition elements have higher enthalpies class 12 chemistry CBSE

What are the advantages of parallel combination over class 12 physics CBSE

What are the causes of overloading class 12 physics CBSE

There is hypertension due to smoking Give scientific class 12 biology CBSE




