Question

The sum of the length, breadth and height of a cuboid is 38 cm and the lengths of its diagonal is 22 cm. Then find the total surface area of the cuboid.

Answer 1

Hint:- Formula for diagonal of the cuboid is $$d=\sqrt{a^2+b^2+c^2}$$ where d is the diagonal and a, b and are the length, breadth and height of the cuboid. And the total surface area of the cuboid is 2(ab + bc + ca). So, let us use the identity of $${\left(a+b+c\right)}^2$$ to find the total surface area of the cuboid.

Complete step-by-step solution -
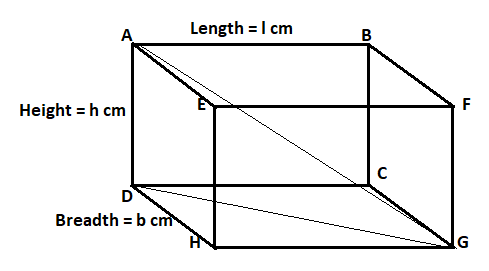
Now as we can see from the above figure that diagonal of the cuboid ABCDHGFE is the line joining the points A and D.
And as we know that a, b, c are the length, breadth and height of any cuboid then its diagonal length must be equal to $$\sqrt{a^2+b^2+c^2}$$.
So, let the length of be AB = DC = EF = HG = l cm
Breadth of the cuboid will be AE = DH = BF = CG = b cm
And, the height of the cuboid will be equal to AD = EH = BC = FG = h cm.
And it is given that the sum of the length, breadth and height of the cuboid is 38 cm.
So, l + b + h = 38 cm (1)
And, $$\sqrt{l^2+b^2+h^2}$$ = 22 cm (2)
On squaring both sides of the above equation. We get,
$$l^2+b^2+h^2=484$$ (3)
Now as we know that the formula for total surface area is 2(ab + bc + ca). So, the total surface area of the above cuboid will be 2(lb + bh + hl).
So, to find the value of the total surface area of the cuboid we must use the identity of $${\left(a+b+c\right)}^2$$. As we know that $${\left(a+b+c\right)}^2=a^2+b^2+c^2+2\left(ab+bc+ca\right)$$.
So, $${\left(l+b+h\right)}^2=l^2+b^2+h^2+2\left(lb+bh+hl\right)$$ (4)
Now putting the value of $$l+b+h$$ and $$l^2+b^2+h^2$$ in equation 4. We get,
$${\left(38\right)}^2=484+2\left(lb+bh+hl\right)$$
Now solving the above equation. We get,
$$1444=484+2\left(lb+bh+hl\right)$$
Subtracting 484 to both sides of the above equation we get,
$$960=2\left(lb+bh+hl\right)$$
As RHS of the above equation is the formula for calculating the total surface area of a cuboid. So, LHS must be equal to the total surface area of the cuboid.
Hence, the total surface area of the cuboid is 960$$c{m}^2$$.
Note:- The main diagonal of the cuboid is one which cuts the cuboid through the centre of it. And length if the diagonal is $$\sqrt{l^2+b^2+h^2}$$ if l, b and h are the dimensions of the cuboid. So, we have to use the formula of calculate the value of $${\left(l+b+h\right)}^2$$ which is $${\left(l+b+h\right)}^2=l^2+b^2+h^2+2\left(lb+bh+hl\right)$$. So, from this identity we can easily get the total surface area of the cuboid value of $$l+b+h$$ is given in the question and $$l^2+b^2+h^2$$ is equal to the square of the diagonal which is also given.