Question

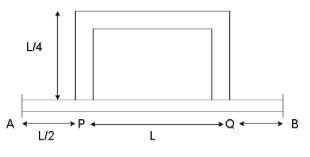
The temperature difference of ${120^ \bullet }C$ is maintained between two ends of a uniform rod AB of length $2L$. Another bent rod PQ of the same cross section as AB and length $\dfrac{{3L}}{2}$, is connected across AB (see figure). In steady state, the temperature difference between P and Q will be close to:
A) ${60^ \bullet }C$
B) ${75^ \bullet }C$
C) ${35^ \bullet }C$
D) ${45^ \bullet }C$

Answer 1

Hint:Temperature difference describes the total amount of internal energy stored in the system. It is the measure of hotness or coldness of a body. If two bodies are at the same temperature then there will be no flow of heat between them.

Complete step by step answer:
Step I:
Given that temperature difference is maintained between the rod AB, this means that there will be some resistance offered to the flow of electrons or charged particles. The resistance of wire AB is given by
$R = \dfrac{{\rho L}}{A}$
Where $\rho $ is the constant of resistivity
$L$ is the length of the rod
$A$ is the area of cross section
In this case, the constant of resistivity and area of the cross section will be the same throughout. Therefore,
$R \propto L$

Step II:
In rod AB, part AP of the rod has length given $\dfrac{L}{2}$
So, resistance will be $ = \dfrac{R}{2}$
Part PQ of the rod has given length$L$
Therefore, resistance $ = R$
Part QB will also have length similar to PA $ = \dfrac{L}{2}$
So, its resistance will be$ = \dfrac{R}{2}$

Step III:
In rod AB all the resistors are connected in series. Therefore equivalent resistance, across PQ is
${({R_{eq}})_{PQ}} = \dfrac{R}{4} + R + \dfrac{R}{4}$
${({R_{eq}})_{PQ}} = \dfrac{{6R}}{4}$
${({R_{eq}})_{PQ}} = \dfrac{{3R}}{2}$

Step IV:
The part PQ of the rod is parallel to the bent rod. Therefore resistance across the bent rod will also be $R$ .
These resistors are parallel. Therefore their resistance will be calculated using the formula,
${R_{eq}} = \dfrac{{{R_1} \times {R_2}}}{{{R_1} + {R_2}}}$
Here ${R_1} = \dfrac{{3R}}{2},{R_2} = R$
${R_{eq}} = \dfrac{{\dfrac{3}{2} \times R}}{{\dfrac{3}{2} + R}}$
${R_{eq}} = \dfrac{3}{2}R \times \dfrac{2}{5}R$
${R_{eq}} = \dfrac{3}{5}{R^2}$

Step V:
Using Ohm’s Law, $V = IR$
$I = \dfrac{V}{R}$
Therefore,
Current through part of wire AB = Current through part of wire PQ
$\dfrac{{{V_B} - {V_A}}}{{\dfrac{R}{2} + \dfrac{R}{2} + \dfrac{3}{5}R}} = \dfrac{{{V_Q} - {V_P}}}{{\dfrac{3}{5}R}}$
$\dfrac{{{V_B} - {V_A}}}{{R + \dfrac{3}{5}R}} = \dfrac{{{V_Q} - {V_P}}}{{\dfrac{3}{5}R}}$
$\dfrac{{{V_B} - {V_A}}}{{\dfrac{8}{5}R}} = \dfrac{{{V_Q} - {V_P}}}{{\dfrac{3}{5}R}}$ ---(i)
Step VI:
Since, current flows in the circuit due to potential difference. But heat flows due to temperature difference. Therefore, in equation (i) replacing potential difference with temperature difference,
$\dfrac{{{T_B} - {T_A}}}{{\dfrac{8}{5}R}} = \dfrac{{{T_Q} - {T_P}}}{{\dfrac{3}{5}R}}$
Given temperature difference, ${T_B} - {T_A} = 120$
Substituting the values,
$\dfrac{{120}}{{\dfrac{8}{5}R}} = \dfrac{{{T_Q} - {T_P}}}{{\dfrac{3}{5}R}}$
${T_Q} - {T_P} = \dfrac{3}{8} \times 120$
${T_Q} - {T_P} = {45^ \bullet }C$

Option D is the right answer.

Note:
It is to be noted that resistance and resistivity are not the same. They are completely different terms. Resistance is the opposition to the flow of current, but resistivity is the resistance offered by the material per unit area of the material.