
The thermo emf of a hypothetical thermocouple varies with the temperature Ѳ of the hot junction as
d) No neutral temperature is possible for this thermocouple
Answer
503.1k+ views
Hint: The above equation for emf of a thermocouple represents an inverted parabola on the y axis if we consider the emf to be on y-axis and the temperature to be on x-axis. The neutral temperature of a thermocouple is the temperature at which the emf of the thermocouple is maximum. For any curve in general if the quantity Y on the y-axis varies with a quantity X on the x-axis then we say that the maximum value of Y is given as
Complete step by step answer:
Let us first see how the emf of a thermocouple varies with temperature.
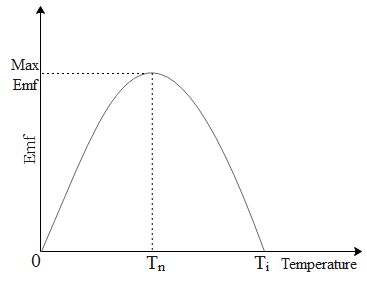
A thermocouple consists of two dissimilar metals connected together to form a closed circuit. One of the junctions is kept at high temperature and the other junction is usually kept at
This thermo emf is maximum at a temperature called as neutral temperature
Let us now use the condition for maximum emf i.e.
Hence differentiating the equation of thermo emf with respect to temperature we get
Since
Further this can be written as,
The neutral temperature of the thermocouple cannot be negative since the cold junction itself is at 0 degree Celsius.
Hence the correct answer is option d.
Note:
If we keep on increasing the temperature even after the thermocouple reaches an inversion temperature the emf will be generated, but it will now be in the opposite direction. Initially if the current was flowing from cold to hot junction, as soon as the temperature crosses the inversion temperature current will flow from hot to cold junction. One more thing we can also generalize is that, if the value of a by b for a thermocouple is positive it cannot have an inversion temperature.
Complete step by step answer:
Let us first see how the emf of a thermocouple varies with temperature.

A thermocouple consists of two dissimilar metals connected together to form a closed circuit. One of the junctions is kept at high temperature and the other junction is usually kept at
This thermo emf is maximum at a temperature called as neutral temperature
Let us now use the condition for maximum emf i.e.
Hence differentiating the equation of thermo emf with respect to temperature we get
Since
Further this can be written as,
The neutral temperature of the thermocouple cannot be negative since the cold junction itself is at 0 degree Celsius.
Hence the correct answer is option d.
Note:
If we keep on increasing the temperature even after the thermocouple reaches an inversion temperature the emf will be generated, but it will now be in the opposite direction. Initially if the current was flowing from cold to hot junction, as soon as the temperature crosses the inversion temperature current will flow from hot to cold junction. One more thing we can also generalize is that, if the value of a by b for a thermocouple is positive it cannot have an inversion temperature.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




