
The three resistors configuration
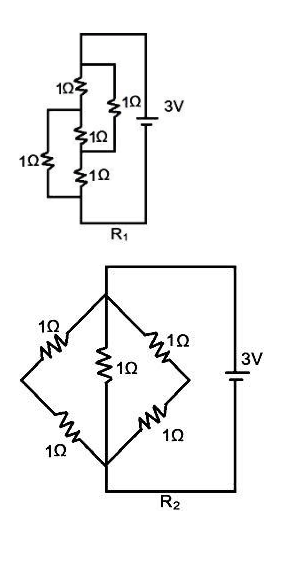
A)
B)
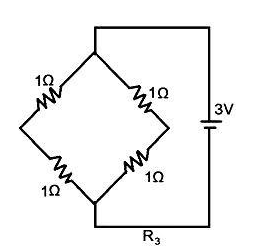
C)
D)


Answer
485.1k+ views
Hint: -Find the equivalent resistances for all the configurations in
Complete Step by Step Solution: -
In the first configuration of
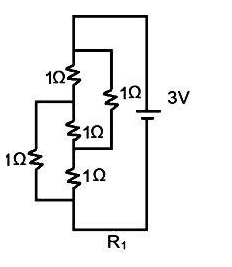
From this configuration, we can conclude that there is an equivalent wheatstone bridge circuit.
Therefore, the equivalent resistance in the configuration
Now, we have to calculate the equivalent resistance in the configuration
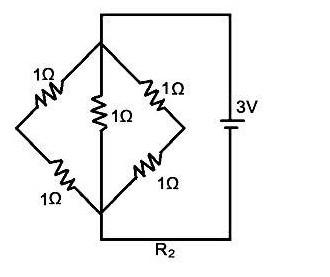
From the above figure, the equivalent resistance can be calculated by –
Therefore, the equivalent resistance in the configuration
Now, we have to calculate the equivalent resistance in the configuration
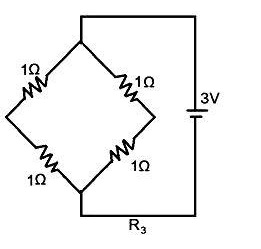
From the above figure, the equivalent resistance for the configuration
Therefore, the equivalent resistance in the configuration
We know that, relationship between power, voltage and resistance is –
So, the power
Power
Power
Now, we have calculated the power for each configuration for
Therefore, we can conclude that –
Hence, the correct option is (C).
Note: -Wheatstone bridge is the circuit which is connected in form of the bridge and is composed of two known resistors, one unknown resistor and one variable resistor connected in the form of a bridge. This bridge gives accurate measurements, so it is most reliable. This bridge is used for the precise measurement of low resistance and is also used to measure inductance, capacitance and impedance by using the variations.
Complete Step by Step Solution: -
In the first configuration of

From this configuration, we can conclude that there is an equivalent wheatstone bridge circuit.
Therefore, the equivalent resistance in the configuration
Now, we have to calculate the equivalent resistance in the configuration

From the above figure, the equivalent resistance can be calculated by –
Therefore, the equivalent resistance in the configuration
Now, we have to calculate the equivalent resistance in the configuration

From the above figure, the equivalent resistance for the configuration
Therefore, the equivalent resistance in the configuration
We know that, relationship between power, voltage and resistance is –
So, the power
Power
Power
Now, we have calculated the power for each configuration for
Therefore, we can conclude that –
Hence, the correct option is (C).
Note: -Wheatstone bridge is the circuit which is connected in form of the bridge and is composed of two known resistors, one unknown resistor and one variable resistor connected in the form of a bridge. This bridge gives accurate measurements, so it is most reliable. This bridge is used for the precise measurement of low resistance and is also used to measure inductance, capacitance and impedance by using the variations.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

What are the major means of transport Explain each class 12 social science CBSE




