
The two coherent sources of equal intensity produce maximum intensity of
a.
b.
c.
d.
Answer
470.7k+ views
1 likes
Hint: To solve the given problem we need to calculate the intensities of the two sources separately and then we need to add the intensities to get the maximum intensity.
Formula used:
The resultant intensity,
Where,
To find the maximum intensity,
Complete step by step answer:
In the question, it is given that the two coherent sources of equal intensity produce maximum intensity of
We can consider the Young’s double slit experiment for better understanding.
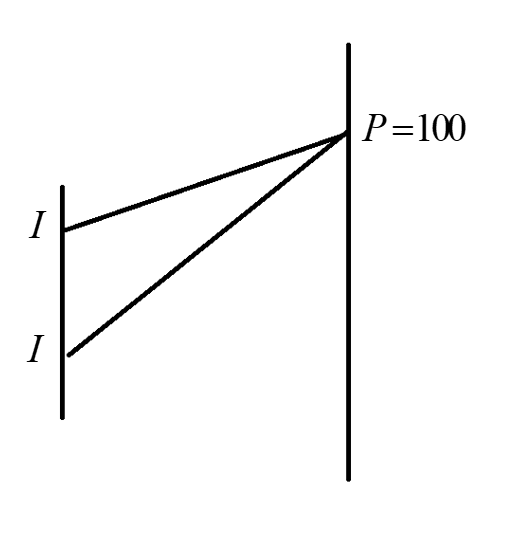
In this diagram, the intensities of the two sources are given and it is represented as
We can consider the maximum intensity. The maximum intensity is given as,
We can simplify the given equation as
In the question it is given that maximum intensity
We have calculated the intensities of the two coherent sources. The intensity value of one source is
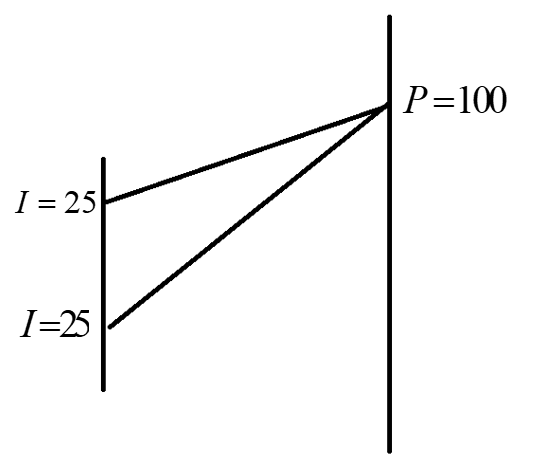
In the question they said that one of the intensities is reduced into
Now we have the value of
From the resultant intensity we can derive the formula for the maximum intensity. That is,
The value of
The resultant intensity is nothing but the maximum intensity, that is
The maximum intensity of the light is given as,
Use addition to simplify the equation to get the maximum intensity.
The intensity of the light at one point is
Hence, the correct answer is option (D).
Note: The light sources that emit the light of same frequency, wavelength are known as coherent sources. Coherent sources are of two types. One is temporal coherence and another one is spatial coherence. When the superimposition of waves occurs, the coherent sources form sustained interference patterns.
Formula used:
The resultant intensity,
Where,
To find the maximum intensity,
Complete step by step answer:
In the question, it is given that the two coherent sources of equal intensity produce maximum intensity of
We can consider the Young’s double slit experiment for better understanding.

In this diagram, the intensities of the two sources are given and it is represented as
We can consider the maximum intensity. The maximum intensity is given as,
We can simplify the given equation as
In the question it is given that maximum intensity
We have calculated the intensities of the two coherent sources. The intensity value of one source is
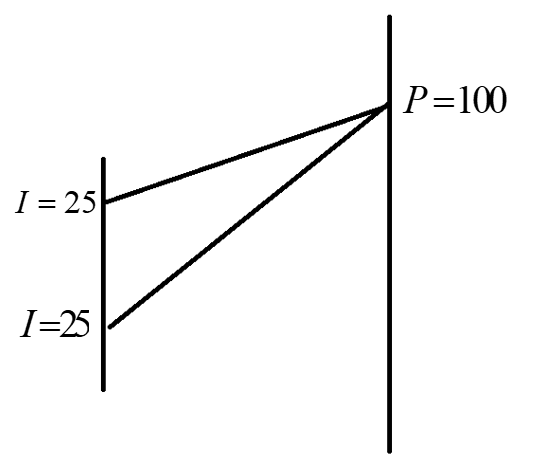
In the question they said that one of the intensities is reduced into
Now we have the value of
From the resultant intensity we can derive the formula for the maximum intensity. That is,
The value of
The resultant intensity is nothing but the maximum intensity, that is
The maximum intensity of the light is given as,
Use addition to simplify the equation to get the maximum intensity.
The intensity of the light at one point is
Hence, the correct answer is option (D).
Note: The light sources that emit the light of same frequency, wavelength are known as coherent sources. Coherent sources are of two types. One is temporal coherence and another one is spatial coherence. When the superimposition of waves occurs, the coherent sources form sustained interference patterns.
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




