Question

The value of acceleration due to gravity:
A.)is same on equator and poles
B.)is least on poles
C.)is least on equator
D.)increases from pole to equator

Answer 1

Hint: Using Newton’s law of gravitation find the equation of gravitational constant and acceleration due to gravity. And according to the formula use the relation between acceleration due ro gravity and radius.

Formula used:
$ g=\quad G\dfrac { M }{ { R }_{ E }^{ 2 } }$

Complete step-by-step answer:
Equation of gravitational constant and acceleration due to gravity can be calculated as shown below:

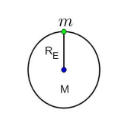
Suppose, a body of mass m is placed on the surface of earth and assumes the shape of the earth to be round. If mass of the earth is M and radius of earth is $ { R }_{ E }$, then by Newton’s law of gravitation we get,
$ F=\quad G\dfrac { Mm }{ { R }_{ E }^{ 2 } }$ …(1)
where, G: Gravitational Constant

Now, from Newton’s Second law of motion,
$ F=\quad mg$ …(2)
where, g: Acceleration due to gravity

From eq.(1) and eq.(2) we get,
$ mg=\quad G\dfrac { Mm }{ { R }_{ E }^{ 2 } }$
$ \therefore \quad g=\quad G\dfrac { M }{ { R }_{ E }^{ 2 } }$
So from the above equation, we can say the value of acceleration due to gravity is least at the maximum radius. Radius is maximum at the equator which means acceleration due to gravity is least at equator.

So, the correct answer is “Option C”.

Note:
As the radius increases acceleration due to gravity decreases. And similarly as the height from the surface of earth increases, acceleration due to gravity decreases. This is given by equation,
$g=\quad G\dfrac { M }{ { ({ R }_{ E }+h) }^{ 2 } } $
where, h: height from the surface of earth