
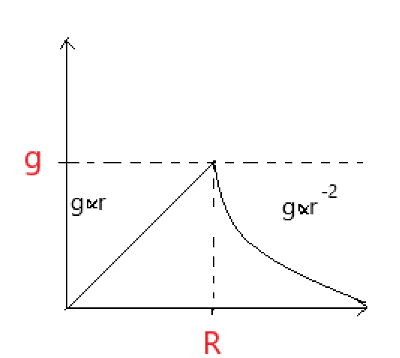
The variation of g with height or depth (r) is shown correctly by the graph in figure (where R is the radius of the earth).
A.
B.
C.
D.




Answer
498.3k+ views
Hint: For this question we start with the equation for g with respect to distance r from the center of the earth with mass M and radius R that is $g = \dfrac{{GM}}{{{r^2}}}$ . Then we find the expression of g when r< R that is ${g^1} = g\left( {1 - \dfrac{{2r}}{R}} \right)$ and when r>R that is ${g^1} = g{\left( {1 + \dfrac{r}{R}} \right)^{ - 2}}$ from these we get the relation of g with respect to r as $ \Rightarrow {g^1} \propto \left( {R - 2r} \right)$ and $ \Rightarrow {g^1} \propto \dfrac{1}{{{r^2}}}$ respectively.
Complete Step-by-Step solution:
We know that the acceleration due to gravity is denoted by the symbol g and is defined as the acceleration of an object because of the force acting on that object by the gravitational field of Earth. This force is written as
$F = mg$
Here m is the mass of the object. If $m = 1$, we get
$F = g$
This also says that g is the force on the unit mass. The g is independent of the mass of the body and can be accurately calculated in various ways.
Now we will try to see the variation of g with respect to height h. So let us assume an object of mass m, then the measure of the gravitational field strength $g$, at a distance r from the center of the earth with mass $M$ is given by:
$g = \dfrac{{GM}}{{{r^2}}}$
Now take the object at the surface of the earth that is r=R as shown in figure 1.
Here R is the radius of the earth. We get
$g = \dfrac{{GM}}{{{R^2}}}$-------------------------------- (1)
Now we assume that the object is at a height H from the surface of the earth as shown in figure 2.

${g^1} = \dfrac{{GM}}{{{{\left( {R + H} \right)}^2}}}$--------------------------- (2)
Now dividing equation (2) by (1) we get
${g^1} = \dfrac{{g{R^2}}}{{{{\left( {R + H} \right)}^2}}}$
$ \Rightarrow {g^1} = \dfrac{g}{{{{\left( {1 + \dfrac{H}{R}} \right)}^2}}}$
$ \Rightarrow {g^1} = g{\left( {1 + \dfrac{H}{R}} \right)^{ - 2}}$-------------------------(3)
This expression is used when $\dfrac{H}{R} \geqslant 1$ that is when the object is at a distance greater than the earth radius.
But when $\dfrac{H}{R} < 1$ we can Apply the binomial expansion and we will get
$ \Rightarrow {g^1} = g\left( {1 - \dfrac{{2H}}{R}} \right)$--------------------------- (4)
From these we can conclude that
Region 1: When $r \leqslant R$ we can write
$ \Rightarrow {g^1} = g\left( {1 - \dfrac{{2r}}{R}} \right)$
$ \Rightarrow {g^1} \propto \left( {R - 2r} \right)$
Region 2: When $r \geqslant R$ we can write
$ \Rightarrow {g^1} = g{\left( {1 + \dfrac{r}{R}} \right)^{ - 2}}$
$ \Rightarrow {g^1} \propto \dfrac{1}{{{r^2}}}$
So the graph we get is shown in figure 3
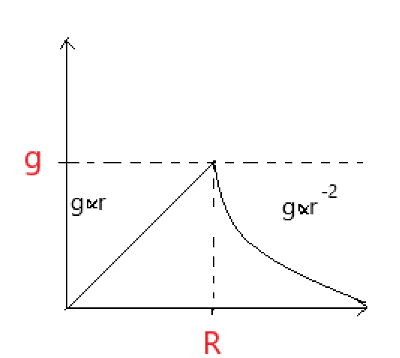
Figure 3
Hence option A is correct.
Note: We can also solve this question in a different way that is we get the expression of g with respect to r when $r < R$ as $g = \dfrac{{GM}}{{{R^3}}}r$ and when $r > R$ we get $g = \dfrac{{GM}}{{{r^2}}}$. By using these also we can plot the required graph.
Complete Step-by-Step solution:
We know that the acceleration due to gravity is denoted by the symbol g and is defined as the acceleration of an object because of the force acting on that object by the gravitational field of Earth. This force is written as
$F = mg$
Here m is the mass of the object. If $m = 1$, we get
$F = g$
This also says that g is the force on the unit mass. The g is independent of the mass of the body and can be accurately calculated in various ways.
Now we will try to see the variation of g with respect to height h. So let us assume an object of mass m, then the measure of the gravitational field strength $g$, at a distance r from the center of the earth with mass $M$ is given by:
$g = \dfrac{{GM}}{{{r^2}}}$
Now take the object at the surface of the earth that is r=R as shown in figure 1.

Figure 1
Here R is the radius of the earth. We get
$g = \dfrac{{GM}}{{{R^2}}}$-------------------------------- (1)
Now we assume that the object is at a height H from the surface of the earth as shown in figure 2.

Figure 2
${g^1} = \dfrac{{GM}}{{{{\left( {R + H} \right)}^2}}}$--------------------------- (2)
Now dividing equation (2) by (1) we get
${g^1} = \dfrac{{g{R^2}}}{{{{\left( {R + H} \right)}^2}}}$
$ \Rightarrow {g^1} = \dfrac{g}{{{{\left( {1 + \dfrac{H}{R}} \right)}^2}}}$
$ \Rightarrow {g^1} = g{\left( {1 + \dfrac{H}{R}} \right)^{ - 2}}$-------------------------(3)
This expression is used when $\dfrac{H}{R} \geqslant 1$ that is when the object is at a distance greater than the earth radius.
But when $\dfrac{H}{R} < 1$ we can Apply the binomial expansion and we will get
$ \Rightarrow {g^1} = g\left( {1 - \dfrac{{2H}}{R}} \right)$--------------------------- (4)
From these we can conclude that
Region 1: When $r \leqslant R$ we can write
$ \Rightarrow {g^1} = g\left( {1 - \dfrac{{2r}}{R}} \right)$
$ \Rightarrow {g^1} \propto \left( {R - 2r} \right)$
Region 2: When $r \geqslant R$ we can write
$ \Rightarrow {g^1} = g{\left( {1 + \dfrac{r}{R}} \right)^{ - 2}}$
$ \Rightarrow {g^1} \propto \dfrac{1}{{{r^2}}}$
So the graph we get is shown in figure 3

Figure 3
Hence option A is correct.
Note: We can also solve this question in a different way that is we get the expression of g with respect to r when $r < R$ as $g = \dfrac{{GM}}{{{R^3}}}r$ and when $r > R$ we get $g = \dfrac{{GM}}{{{r^2}}}$. By using these also we can plot the required graph.
Recently Updated Pages
What percentage of the area in India is covered by class 10 social science CBSE

The area of a 6m wide road outside a garden in all class 10 maths CBSE

What is the electric flux through a cube of side 1 class 10 physics CBSE

If one root of x2 x k 0 maybe the square of the other class 10 maths CBSE

The radius and height of a cylinder are in the ratio class 10 maths CBSE

An almirah is sold for 5400 Rs after allowing a discount class 10 maths CBSE

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

What constitutes the central nervous system How are class 10 biology CBSE

Write a letter to the principal requesting him to grant class 10 english CBSE

Explain the Treaty of Vienna of 1815 class 10 social science CBSE




