
The
(A)
(B)
(C)
(D)
Answer
467.4k+ views
Hint: The incenter of a triangle is the center of its inscribed circle. Here, the mid points of the sides of the triangle are given. In order to find the incenter of any triangle, we need the coordinates of the triangle and the length of the corresponding sides respectively. So, we need to find the coordinates of the triangle and the length of its sides. The formula of incenter of any triangle is given below:
Similarly,
Where,
Complete solution step by step:
After plotting the points given in the question, we get a triangle with coordinates
Similarly,
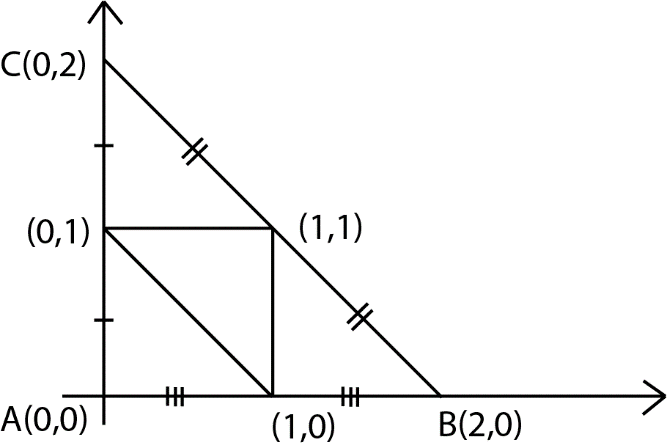
From
We got the coordinates
Distance between any two points
Length of side
Length of side
Now, we got all the coordinates and the length of all the sides of the triangle.
We have the formula of incenter:
Where,
Here,
Putting all the values in the formula,
On rationalizing
Therefore, the correct answer is option (A).
Note:
In this question, we were asked to find the
Similarly,
Where,
Complete solution step by step:
After plotting the points given in the question, we get a triangle with coordinates
Similarly,

From
We got the coordinates
Distance between any two points
Length of side
Length of side
Now, we got all the coordinates and the length of all the sides of the triangle.
We have the formula of incenter:
Where,
Here,
Putting all the values in the formula,
On rationalizing
Therefore, the correct answer is option (A).
Note:
In this question, we were asked to find the
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹42,330 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Whom did king Ashoka send to Sri Lanka to spread Buddhism class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

How many crores make 10 million class 7 maths CBSE

AIM To prepare stained temporary mount of onion peel class 7 biology CBSE

Find HCF and LCM of 120 and 144 by using Fundamental class 7 maths CBSE





