
There are four concentric shells A, B, C and D of radii
(A)
(B)
(C)
(D)
Answer
479.7k+ views
1 likes
Hint
We need to find the potential at shell C and then equate that value to zero. From there we can find the charge induced on C. Therefore, by calculating the value of the potential at the shells A and C and we can find the difference in their potential.
Formula Used: In this solution, we will be using the following formula
where
Complete step by step answer
In this case there are 4 concentric shells A, B, C, and D of radius
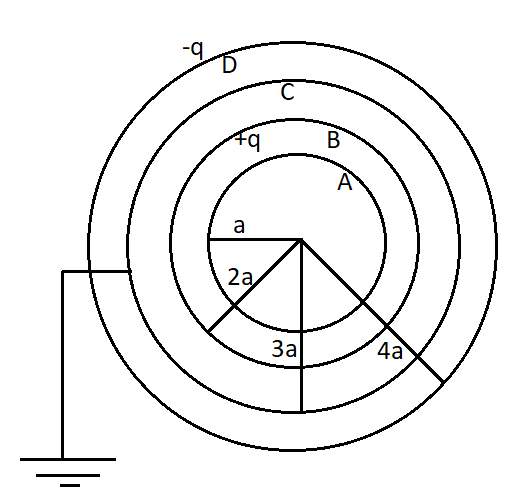
The electric potential due to a charge can be given by the formula,
Now the potential on the shell C due to the charges placed on the shells D end B is,
where
And the potential on the surface of A is,
Now the shell C is grounded. So the potential on C will be 0. That is,
So equating the equation we get,
We can cancel the
So we get,
Therefore taking the term containing
On the R.H.S taking LCM, we find
On doing the calculation we get
Now putting this value of
On doing the LCM and calculating further we get3
Hence we get the value of the potential at the surface of A as,
Since
Therefore
This is the difference in potential. So the correct option is D.
Note
The electric potential at a point in an electric field is the amount of work that is done in bringing a unit positive charge from infinity to that point. And when a body is charged it can attract and repulse an oppositely charged body. This shows the ability of a charged body to do work. This ability is called the potential of that body.
We need to find the potential at shell C and then equate that value to zero. From there we can find the charge induced on C. Therefore, by calculating the value of the potential at the shells A and C and we can find the difference in their potential.
Formula Used: In this solution, we will be using the following formula
where
Complete step by step answer
In this case there are 4 concentric shells A, B, C, and D of radius

The electric potential due to a charge can be given by the formula,
Now the potential on the shell C due to the charges placed on the shells D end B is,
where
And the potential on the surface of A is,
Now the shell C is grounded. So the potential on C will be 0. That is,
So equating the equation we get,
We can cancel the
So we get,
Therefore taking the term containing
On the R.H.S taking LCM, we find
On doing the calculation we get
Now putting this value of
On doing the LCM and calculating further we get3
Hence we get the value of the potential at the surface of A as,
Since
Therefore
This is the difference in potential. So the correct option is D.
Note
The electric potential at a point in an electric field is the amount of work that is done in bringing a unit positive charge from infinity to that point. And when a body is charged it can attract and repulse an oppositely charged body. This shows the ability of a charged body to do work. This ability is called the potential of that body.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




