
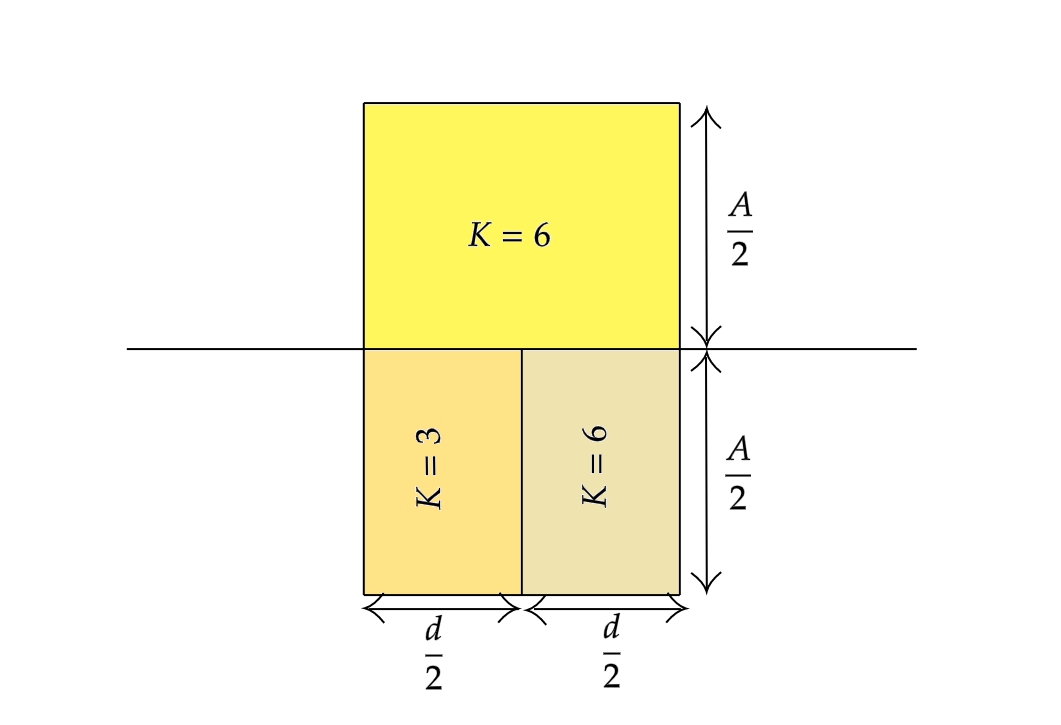
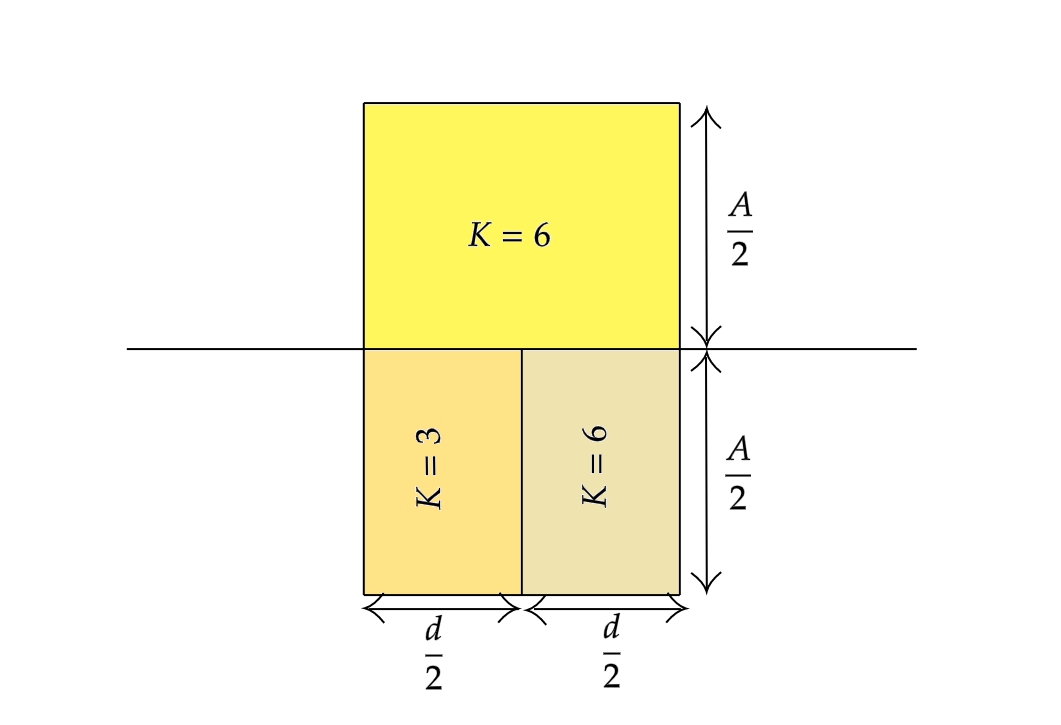
Three different dielectrics are filled in a parallel plate capacitor as shown. What should be the dielectric constant of a material, which when fully filled between the plates produces the same capacitance?

Answer
402.6k+ views
Hint: Whenever a dielectric is inserted between a parallel plate of a capacitor then the new value of the capacitor increases by k time the original capacitor. And if more than one dietetic material is inserted in between the parallel plated then each medium will act as a separate capacitor. Now using this concept first convert each dielectric into a separate capacitor so as to analyze their connection that they are in series or parallel. Now find the equivalent capacitor of the three dielectric materials and equate it with the original capacitance.
Complete answer:
We know that capacitance of a capacitor is given by,
Where,
A is the area between the two parallel plates.
Distance between the two parallel plates is represented as d.
And
This is the case when it is between the two parallel plates.
But in this case three dielectric constants are inserted and in total let the dielectric constant be K which is always greater than one.
Therefore the new capacitance will become,
Which is K time greater
Now from the above figure we can divide the capacitor into three different capacitors having different dielectric constant.
Case i:
Now on simplifying we will get,
Case II:
In series with
Now on simplifying we will get,
Case III:
In parallel with
Now on simplifying we will get,
Now we can draw,
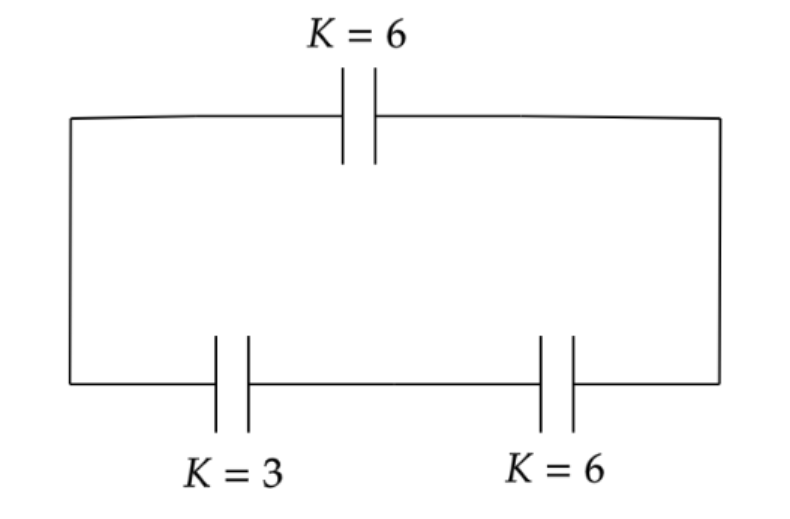
Now the equivalent capacitance from the above diagram will be,
Putting the values we will get,
Cancelling the common terms qe will get,
Parallel capacitance are added,
As per the problem now equating this equivalent capacitance with
Putting the respective values we will get,
Cancelling the given terms we will get,
Therefore the correct option is
Note:
Remember that different materials have different dielectric constants like a perfectly conducting material has a dielectric constant of infinity while a perfectly insulating parallel has dielectric constant as zero. And note that while solving this type of problem first convert the dielectric into a separate category to solve the problem easily.
Complete answer:
We know that capacitance of a capacitor is given by,
Where,
A is the area between the two parallel plates.
Distance between the two parallel plates is represented as d.
And
This is the case when it is between the two parallel plates.
But in this case three dielectric constants are inserted and in total let the dielectric constant be K which is always greater than one.
Therefore the new capacitance will become,
Which is K time greater

Now from the above figure we can divide the capacitor into three different capacitors having different dielectric constant.
Case i:
Now on simplifying we will get,
Case II:
In series with
Now on simplifying we will get,
Case III:
In parallel with
Now on simplifying we will get,
Now we can draw,

Now the equivalent capacitance from the above diagram will be,
Putting the values we will get,
Cancelling the common terms qe will get,
Parallel capacitance are added,
As per the problem now equating this equivalent capacitance with
Putting the respective values we will get,
Cancelling the given terms we will get,
Therefore the correct option is
Note:
Remember that different materials have different dielectric constants like a perfectly conducting material has a dielectric constant of infinity while a perfectly insulating parallel has dielectric constant as zero. And note that while solving this type of problem first convert the dielectric into a separate category to solve the problem easily.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




