Question

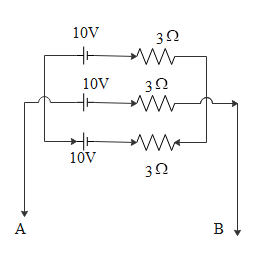
Three identical cells are connected in parallel across AB. Net emf across AB is

Answer 1

Hint: Given three identical cells connected in parallel having each internal resistance of 3 ohm. When we connect the voltage sources having the same emf which are connected in parallel and then each one is connected to the load resistance. Then the total emf will be the same as that of individual emfs.

Complete step-by-step solution:
Generally with n batteries ${{\varepsilon }_{i}}$with internal resistances ${{R}_{i}}$ is connected in parallel. Then we can calculate the equivalent emf using the equation,
${{\varepsilon }_{eq}}=\dfrac{\dfrac{{{\varepsilon }_{1}}}{{{R}_{1}}}+\dfrac{{{\varepsilon }_{2}}}{{{R}_{2}}}+\dfrac{{{\varepsilon }_{3}}}{{{R}_{3}}}+.........+\dfrac{{{\varepsilon }_{n}}}{{{R}_{n}}}}{\dfrac{1}{{{R}_{1}}}+\dfrac{1}{{{R}_{2}}}+\dfrac{1}{{{R}_{3}}}+........+\dfrac{1}{{{R}_{n}}}}$
Here we have three sources of emfs and three resistance which are connected in parallel. Hence the equation becomes,
${{\varepsilon }_{eq}}=\dfrac{\dfrac{{{\varepsilon }_{1}}}{{{R}_{1}}}+\dfrac{{{\varepsilon }_{2}}}{{{R}_{2}}}+\dfrac{{{\varepsilon }_{3}}}{{{R}_{3}}}}{\dfrac{1}{{{R}_{1}}}+\dfrac{1}{{{R}_{2}}}+\dfrac{1}{{{R}_{3}}}}$
Also given that,
${{\varepsilon }_{1}}={{\varepsilon }_{2}}={{\varepsilon }_{3}}=10V$
${{R}_{1}}={{R}_{2}}={{R}_{3}}=3\Omega $
Substituting these values in the above equation we get,
\[{{\varepsilon }_{eq}}=\dfrac{\dfrac{10}{3}+\dfrac{10}{3}+\dfrac{10}{3}}{\dfrac{1}{3}+\dfrac{1}{3}+\dfrac{1}{3}}\]
Thus we get,
\[\begin{align}
  & {{\varepsilon }_{eq}}=\dfrac{30}{3} \\
 & \Rightarrow {{\varepsilon }_{eq}}=10V \\
\end{align}\]
Therefore we can say that the equivalent emf in a parallel circuit is equal to the individual emf of the battery.

Note: The houses are wired in parallel and never use series connection because in the case of a parallel circuit the loads can operate with respect to the device we use. Thus we use series connections rare for household wirings. Since in series the total voltage is divided equally among all we will get the required voltage for a particular device. And also when we connect the voltage sources having the same emf which are connected in parallel and then each one is connected to the load resistance. Then the total emf will be the same as that of individual emfs.