Question

What is the total emf, when three cells of emfs 2 V, 2 V, 2 V are connected in parallel?
${\text{A}}{\text{.}}$ 4 V
${\text{B}}{\text{.}}$ 1 V
${\text{C}}{\text{.}}$ 2 V
${\text{D}}{\text{.}}$ 5 V

Answer 1

Hint: Here, we will proceed by defining the term electromotive force (emf). Then, we will write down the relation between the terminal potential difference (V) and emf (E). Then, we will write the important concept when cells of the same emfs are connected in parallel.

Complete Step-by-Step solution:

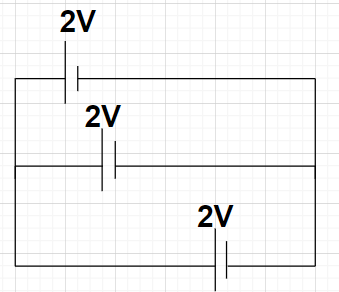
Given, emf of the first cell = emf of the second cell = emf of the third cell = 2 V
EMF or Electromotive force is defined as the potential difference that develops within an open circuit between the two terminals of a battery. The emf is the theoretical difference between the positive terminal anode and the negative terminal cathode when no current flows through it. The emf tests the energy that is transferred in the cell or a battery to the charge borne. It is the energy in joules which is divided by the coulombs charge. The emf serves as the triggering force for flow of the current.
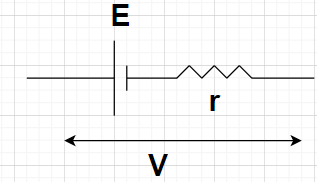
When internal resistance is considered, then the terminal potential difference is given by
V = E – Ir where V denotes the terminal potential difference, E denotes the emf, I denotes the current and r denotes the internal resistance
Usually internal resistance of a cell is not considered because ε >> Ir. The value of internal resistance changes from cell to cell.
In this case also, there is no mentioning of internal resistance of the cells, So, we will neglect it and we get consider that the terminal potential difference between the terminals is equal to the electromotive force (emf).
When n number of cells each of emf E are connected in parallel with each other, then the net equivalent emf of the system will be equal to the individual emf E
Clearly, the emfs of all the three cells which are connected in parallel are equal. So, the total emf of the circuit will be equal to the individual emf of the cell.
Therefore, the required total emf of the system will be equal to 2 V.
Hence, option C is correct.

Note- Cells are joined end-to - end in sequence, so that the same current flows through each cell. If the cells are connected in series the battery emf is equal to the sum of the individual cells emf. The overall emf of the battery E = ${{\text{E}}_1} + {{\text{E}}_2} + {{\text{E}}_3} + {{\text{E}}_4} + ... + {{\text{E}}_{\text{n}}}$ where ${{\text{E}}_1}$, ${{\text{E}}_2}$, ${{\text{E}}_3}$,…, ${{\text{E}}_{\text{n}}}$ are the emfs of individual cells.