
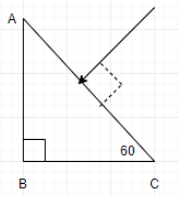
Trace the path of a ray of light passing through a glass prism ABC as shown in the figure. If the refractive index of glass is

Answer
464.1k+ views
1 likes
Hint:This is a case of the occurrence of refraction, where the light bends when it travels to a medium with a different value of refractive index. In a prism, the deviation takes place twice. Hence to trace the path of light that passes through the prism, we should note the fact that the refracting surfaces of the prism aren’t parallel to each other.
Formulas used:
Formula for Snell’s law:
Where
Complete step by step answer:
Refractive index of a medium can be defined as the phenomenon where light bends when it travels from one medium to another, with variation in refractive index. Refraction in a glass prism does not involve parallel surfaces. Thus, refraction occurs twice in a prism. The first time is when the ray enters the prism and the second time when the ray emerges out of the prism.
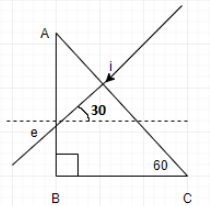
We know that according to Snell’s law,
Or
At the surface AB,
Thus we apply the Snell’s law again to find the angle of emergence e
Hence the value of the angle of emergence is
Note:Refraction through a prism is entirely different from that of a glass slab because here, the incident light is not parallel to the emergent light and it bends towards the base of the prism. Also, the phenomenon of dispersion takes place in the glass prism and not in the glass slab because of the complete variation in the construction and geometry of both of those. Hence, the light emerges out in totally different methods and the corresponding questions are to be approached in different manners because through the material is same, the construction is different.
Formulas used:
Formula for Snell’s law:
Where
Complete step by step answer:
Refractive index of a medium can be defined as the phenomenon where light bends when it travels from one medium to another, with variation in refractive index. Refraction in a glass prism does not involve parallel surfaces. Thus, refraction occurs twice in a prism. The first time is when the ray enters the prism and the second time when the ray emerges out of the prism.

We know that according to Snell’s law,
Or
At the surface AB,
Thus we apply the Snell’s law again to find the angle of emergence e
Hence the value of the angle of emergence is
Note:Refraction through a prism is entirely different from that of a glass slab because here, the incident light is not parallel to the emergent light and it bends towards the base of the prism. Also, the phenomenon of dispersion takes place in the glass prism and not in the glass slab because of the complete variation in the construction and geometry of both of those. Hence, the light emerges out in totally different methods and the corresponding questions are to be approached in different manners because through the material is same, the construction is different.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write a short note on Franklands reaction class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE




