
Two concentric coils each of radius equal to 2
A.
B.
C.
D.
Answer
512.7k+ views
Hint – For these types of questions, we must know the basic formulae of the magnetic chapter and how to apply in different situations of the coils i.e. if they are parallel or perpendicular.
Formula used – 1)
2)
Complete step-by-step solution -
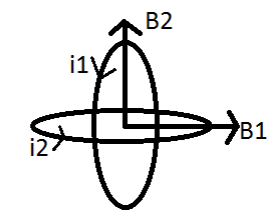
Given that,
We know that,
And
According to the flow of current, the magnetic fields will be perpendicular to the plane of the respective rings.
The value of
On putting the value of radius and
Therefore,
Hence the correct answer to this question is
Hence the correct option is C.
Note – In solving this question we must know the application of formula according to the configuration of the two coils and the calculation mistake should be avoided. Since students generally make calculation mistakes in these questions. We advise the students to remember the formula of the magnetic field for rings at the axis and at the center. Remembering derivation will give you a brief knowledge to calculate the magnetic field for any other shape regarding a ring.
Formula used – 1)
2)
Complete step-by-step solution -

Given that,
We know that,
And
According to the flow of current, the magnetic fields will be perpendicular to the plane of the respective rings.
The value of
On putting the value of radius and
Therefore,
Hence the correct answer to this question is
Hence the correct option is C.
Note – In solving this question we must know the application of formula according to the configuration of the two coils and the calculation mistake should be avoided. Since students generally make calculation mistakes in these questions. We advise the students to remember the formula of the magnetic field for rings at the axis and at the center. Remembering derivation will give you a brief knowledge to calculate the magnetic field for any other shape regarding a ring.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write a short note on Franklands reaction class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE




