
Two parallel infinite line charges with linear charge densities
Answer
490.8k+ views
Hint: Electric field for a line charge can be calculated using Coulomb’s Law, which can help to get the electric force between the two line charges and since the details regarding the linear charge densities and the distance between them are given, then to get the net electric field between the two-line charges, we just need to add the electric field of both line charges individually.
Formula used:
Complete step by step answer:
According to the question, the magnitude of the charge density is
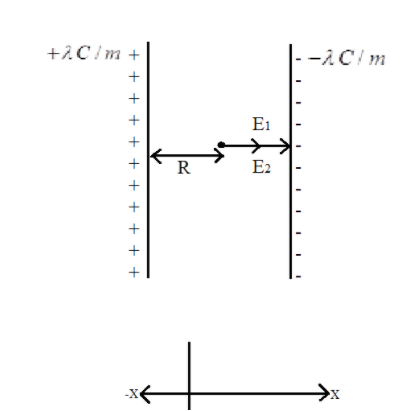
So, the electric field due to line charge
And, the electric field due to line charge
Hence, the electric field mid-way between the two-line charges can be written as:
Substituting the value of both the line charge electric field, we get:
Note:
It’s better to construct a diagram for the details given in the question, which helps to understand the problem easily and also shows a way of getting the solution. In this question, after marking the details given in a diagram, it was easy to find the net electric field by just placing the values in the formula.
Formula used:
Complete step by step answer:
According to the question, the magnitude of the charge density is

So, the electric field due to line charge
And, the electric field due to line charge
Hence, the electric field mid-way between the two-line charges can be written as:
Substituting the value of both the line charge electric field, we get:
Note:
It’s better to construct a diagram for the details given in the question, which helps to understand the problem easily and also shows a way of getting the solution. In this question, after marking the details given in a diagram, it was easy to find the net electric field by just placing the values in the formula.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




