
Two parallel plate capacitors of capacitance
Answer
442.5k+ views
1 likes
Hint :Here, Capacitors are the components in the electric circuit which stores charge according to the capacity of the capacitor. The combinatorial formula for calculating the capacitance of capacitors connected in parallel is similar to that of the resistance equivalent we calculate in series. And vice versa. Use the proper circuit diagram according to the conditions given in the question.
Complete Step By Step Answer:
According to the condition given in the question let us draw a proper circuit diagram containing two capacitors in the parallel combination and a potential is applied to them as shown in the figure
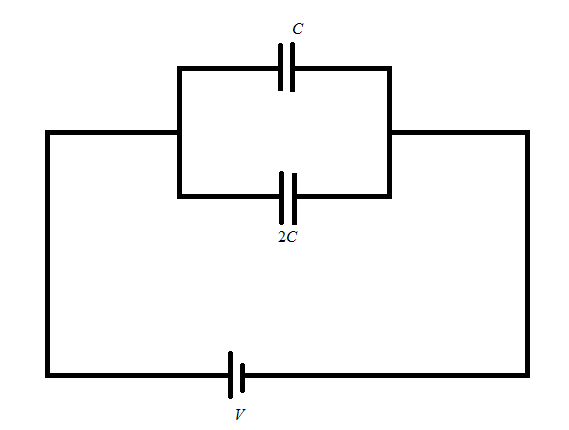
First, let the charge on capacitor
And charge on capacitor
Also, it is given that the capacitor with capacitance
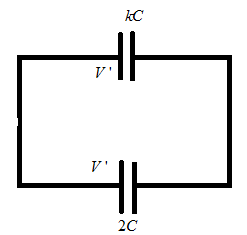
Now, when dielectric is filled in capacitor
And similarly for capacitor
From figures
That is why, we have to use the formula for conservation of charges in capacitors having same capacitance but different voltage across them to calculate new potential difference.
The new voltage across the capacitor
Note :
We know that the definition says that the charges on a capacitor is the product of the capacity of the capacitor and the potential difference across it i.e.
Complete Step By Step Answer:
According to the condition given in the question let us draw a proper circuit diagram containing two capacitors in the parallel combination and a potential is applied to them as shown in the figure

First, let the charge on capacitor
And charge on capacitor
Also, it is given that the capacitor with capacitance

Now, when dielectric is filled in capacitor
And similarly for capacitor
From figures
That is why, we have to use the formula for conservation of charges in capacitors having same capacitance but different voltage across them to calculate new potential difference.
The new voltage across the capacitor
Note :
We know that the definition says that the charges on a capacitor is the product of the capacity of the capacitor and the potential difference across it i.e.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




