Question

Two tangents on a parabola are $x-y=0$ and $x+y=0$. If $\left( 2,3 \right)$ is the focus of the parabola, then find the equation of the tangent at the vertex.
(a) $4x-6y+5=0$
(b) $4x-6y+3=0$
(c) $4x-6y+1=0$
(d) $4x-6y+\dfrac{3}{2}=0$

Answer 1

Hint: We start solving the problem by recalling that the foot of perpendicular from focus to any tangent of the parabola lies on the tangent at its vertex. We then find the foot of perpendiculars of the focus on to the given tangents by first finding the lines perpendicular to tangents and passing through focus and then finding the intersection of them. We then find the equation of the tangent at the vertex which is passing through the obtained foot of perpendiculars to get the required answer.

Complete step by step answer:
According to the problem, we are given that two tangents on a parabola are $x-y=0$ and $x+y=0$. We need to find the equation of the tangent at the vertex of the parabola if $\left( 2,3 \right)$ is the focus of the parabola.
We know that the foot of perpendicular from focus to any tangent of the parabola lies on the tangent at its vertex.

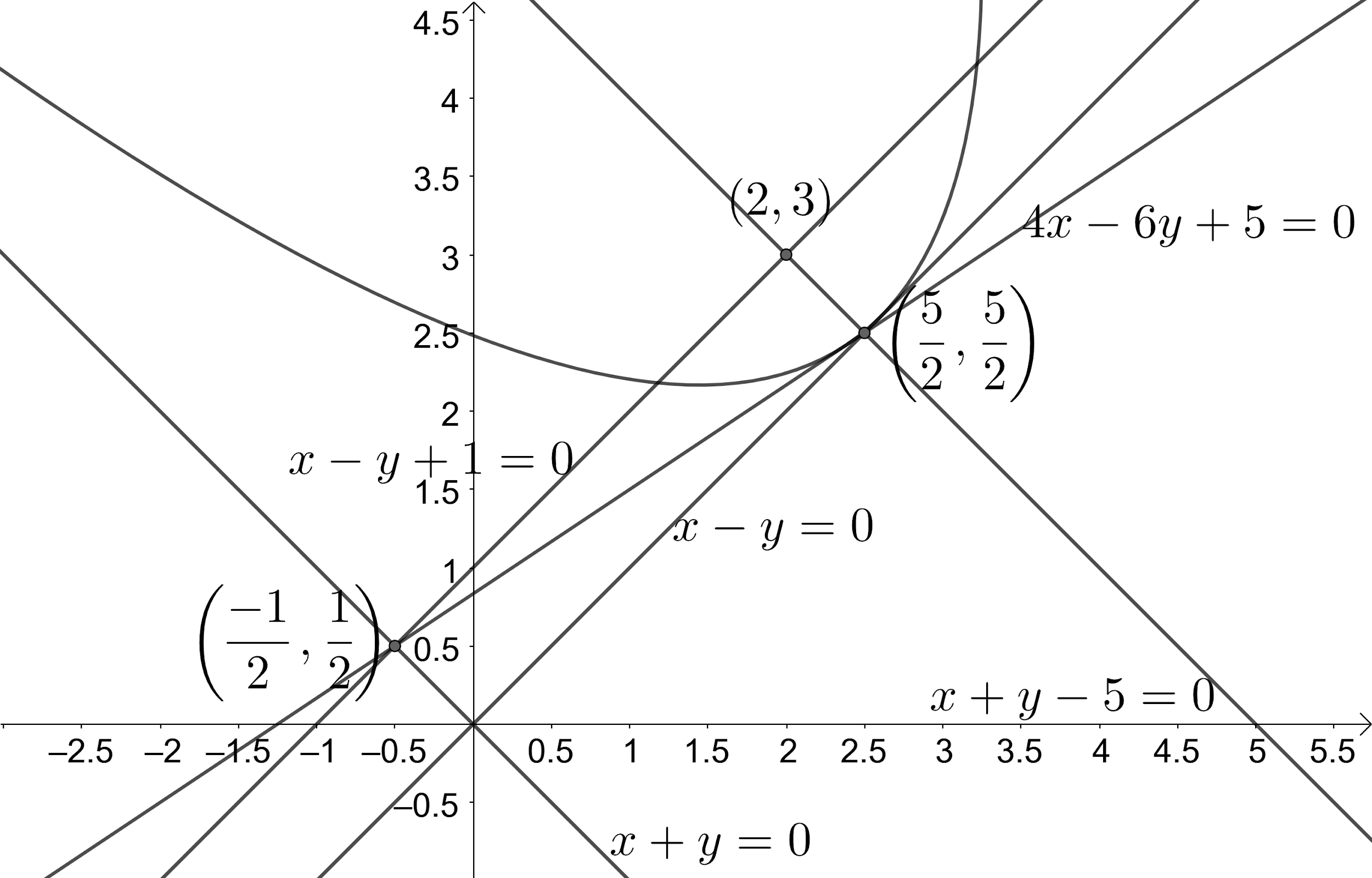
Let us find the equation of the line perpendicular to the tangent $x-y=0$ and passing through $\left( 2,3 \right)$.
We have given the line $x-y=0\Leftrightarrow y=x$. Comparing this with $y=mx$, we get the slope of the tangent as 1.
We know that the product of the slopes of two perpendicular lines is -1. Let us assume the slope of the required line be ${{m}_{1}}$.
So, we get $1\times {{m}_{1}}=-1\Leftrightarrow {{m}_{1}}=-1$.
We know that the equation of the line passing through the point $\left( a,b \right)$ and having slope m is $y-{{y}_{1}}=m\left( x-{{x}_{1}} \right)$.
So, we get the equation as $y-3=-1\left( x-2 \right)$.
$\Rightarrow y-3=-x+2$.
$\Rightarrow x+y-5=0$. Now, let us find the intersection point of $x-y=0$ and $x+y-5=0$.
So, we get $x+x-5=0$.
$\Rightarrow 2x-5=0$.
$\Rightarrow x=\dfrac{5}{2}$.
So, we have found the intersection point as $A\left( \dfrac{5}{2},\dfrac{5}{2} \right)$ ---(1).
Let us find the equation of the line perpendicular to the tangent $x+y=0$ and passing through $\left( 2,3 \right)$.
We have given the line $x+y=0\Leftrightarrow y=-x$. Comparing this with $y=mx$, we get the slope of the tangent as -1.
We know that the product of the slopes of two perpendicular lines is -1. Let us assume the slope of the required line be ${{m}_{2}}$.
So, we get $-1\times {{m}_{2}}=-1\Leftrightarrow {{m}_{2}}=1$.
We know that the equation of the line passing through the point $\left( a,b \right)$ and having slope m is $y-{{y}_{1}}=m\left( x-{{x}_{1}} \right)$.
So, we get the equation as $y-3=1\left( x-2 \right)$.
$\Rightarrow y-3=x-2$.
$\Rightarrow x-y+1=0$. Now, let us find the intersection point of $x+y=0$ and $x-y+1=0$.
So, we get $-y-y+1=0$.
$\Rightarrow -2y+1=0$.
$\Rightarrow y=\dfrac{1}{2}$.
We have $x=-y\Leftrightarrow x=\dfrac{-1}{2}$.
So, we have found the intersection point as $B\left( \dfrac{-1}{2},\dfrac{1}{2} \right)$ ---(2).
Now, we need to find the equation of the lines passing through points $A\left( \dfrac{5}{2},\dfrac{5}{2} \right)$ and $B\left( \dfrac{-1}{2},\dfrac{1}{2} \right)$.
We know that the equation of the line passing through the points $\left( {{a}_{1}},{{b}_{1}} \right)$ and $\left( {{a}_{2}},{{b}_{2}} \right)$ is $y-{{b}_{1}}=\left( \dfrac{{{b}_{2}}-{{b}_{1}}}{{{a}_{2}}-{{a}_{1}}} \right)\times \left( x-{{a}_{1}} \right)$.
So, we get equation of the tangent as $y-\dfrac{5}{2}=\left( \dfrac{\dfrac{1}{2}-\dfrac{5}{2}}{\dfrac{-1}{2}-\dfrac{5}{2}} \right)\times \left( x-\dfrac{5}{2} \right)$.
$\Rightarrow y-\dfrac{5}{2}=\left( \dfrac{-\dfrac{4}{2}}{-\dfrac{6}{2}} \right)\times \left( x-\dfrac{5}{2} \right)$.
$\Rightarrow y-\dfrac{5}{2}=\left( \dfrac{2}{3} \right)\times \left( x-\dfrac{5}{2} \right)$.
$\Rightarrow 3y-\dfrac{15}{2}=2x-\dfrac{10}{2}$.
$\Rightarrow 2x-3y+\dfrac{5}{2}=0$.
$\Rightarrow 4x-6y+5=0$.
We have found the equation of the tangent at the vertex as $4x-6y+5=0$.

So, the correct answer is “Option a”.

Note: We can see that the given problem contains a huge amount of calculation so, we need to perform each step carefully in order to avoid confusion and calculation mistakes. We can prove the result that the foot of perpendicular from focus to any tangent of the parabola lies on the tangent at its vertex for the standard equation of parabola which can be used for any parabola. Similarly, we can expect problems to find the axis and equation of the parabola using the obtained equation of tangent.