
Two tetrahedral dice with faces marked 1, 2, 3 and 4 are thrown. The score obtained is the sum of the numbers on the bottom face. Calculate the probability distribution for the score obtained and how?
Answer
403.8k+ views
Hint: A tetrahedral is a 3-Dimensional object which has four faces. So, we use this concept and concept of probability to solve this problem. A probability distribution table gives us the information about probabilities of happening of different possibilities of an event. So using probability distribution we will get the required solution.
Complete step by step answer:
In mathematics, probability is defined as the occurrence of a random event. It is also defined as the ratio of number of favorable outcomes to total number of outcomes.
So,
If the probability of an event is 0, then the event doesn’t happen.
If the probability of an event is 1, then it will happen for sure.
Now if two tetrahedral dice are rolled at a time, we get the possible outcomes as
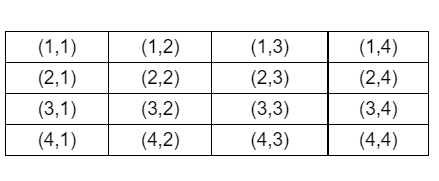
There are a total 16 possible outcomes.
So, if we add the numbers on the bottom faces of the tetrahedral, we get the scores.
And the least score possible is
And the highest score is
So, if we roll two tetrahedral dice, then the possible scores that we can get are
So, the probability of getting score 2 is
So,
Probability of getting score 3 is
So,
Probability of getting score 4 is
So,
Probability of getting a score 5 is
So,
Probability of getting a score 6 is
So,
Probability of getting a score 7 is
So,
Probability of getting score 8 is
So,
On tabulating these values, we get probability distribution table as
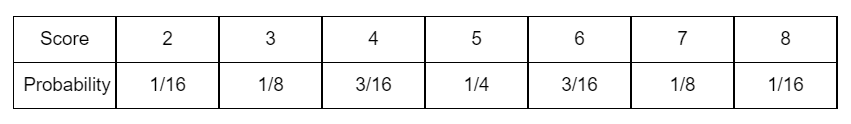
Note:
Make a note that the probability always lies in the range [0,1]. So, if you get a negative value or a value greater than 1, then you have made a mistake in your solution. And also remember that the sum of probabilities of random possibilities of an event is always equal to 1.
So, here,
Complete step by step answer:
In mathematics, probability is defined as the occurrence of a random event. It is also defined as the ratio of number of favorable outcomes to total number of outcomes.
So,
If the probability of an event is 0, then the event doesn’t happen.
If the probability of an event is 1, then it will happen for sure.
Now if two tetrahedral dice are rolled at a time, we get the possible outcomes as

There are a total 16 possible outcomes.
So, if we add the numbers on the bottom faces of the tetrahedral, we get the scores.
And the least score possible is
And the highest score is
So, if we roll two tetrahedral dice, then the possible scores that we can get are
So, the probability of getting score 2 is
So,
Probability of getting score 3 is
So,
Probability of getting score 4 is
So,
Probability of getting a score 5 is
So,
Probability of getting a score 6 is
So,
Probability of getting a score 7 is
So,
Probability of getting score 8 is
So,
On tabulating these values, we get probability distribution table as

Note:
Make a note that the probability always lies in the range [0,1]. So, if you get a negative value or a value greater than 1, then you have made a mistake in your solution. And also remember that the sum of probabilities of random possibilities of an event is always equal to 1.
So, here,
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Basicity of sulphurous acid and sulphuric acid are

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




