
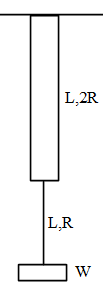
Two wires of the same material (young’s modulus Y) and same length L but radii R and 2R respectively are joined end to end and a weight W is suspended from the combination as shown in the figure. The elastic potential energy in the system in equilibrium is:
A) $\dfrac{{3{W^2}L}}{{4\pi {R^2}Y}}$.
B) $\dfrac{{3{W^2}L}}{{8\pi {R^2}Y}}$.
C) $\dfrac{{5{W^2}L}}{{8\pi {R^2}Y}}$.
D) $\dfrac{{{W^2}L}}{{\pi {R^2}Y}}$.
Answer
147.6k+ views
Hint: The potential energy is the energy which is saved inside the wire and is caused due to the elongation of the wire. The young’s modulus of a material is defined as the ratio of the stress and strain and it is constant till the proportional limit.
Formula used: The formula of the potential energy is given by,
$ \Rightarrow E = \dfrac{{{F^2}L}}{{2AY}}$
Where force is F, the original length is L, the area is A and Young’s modulus is Y.
Complete step by step solution:
It is given in the problem that the two wires of the same material (young’s modulus Y) and same length L but radii R and 2R respectively are joined end to end and a weight W is suspended from the combination and we need to find the elastic potential energy in the system in equilibrium condition.
The formula of the potential energy is given by,
$ \Rightarrow E = \dfrac{{{F^2}L}}{{2AY}}$
Where force is F, the original length is L, the area is A and Young’s modulus is Y.
The potential energy of the wire is equal to,
$ \Rightarrow E = \dfrac{{{F^2}L}}{{2AY}}$
The elongation is taking place in the two parts of the wires.
The potential energy of the system is equal to,
$ \Rightarrow E = \dfrac{{{W^2}L}}{{2{A_1}Y}} + \dfrac{{{W^2}L}}{{2{A_2}Y}}$
$ \Rightarrow E = \dfrac{{{W^2}L}}{{2\left( {\pi {R^2}} \right)Y}} + \dfrac{{{W^2}L}}{{2\pi {{\left( {2R} \right)}^2}Y}}$
$ \Rightarrow E = \dfrac{{{W^2}L}}{{2\pi {R^2}Y}} + \dfrac{{{W^2}L}}{{8\pi {R^2}Y}}$
$ \Rightarrow E = \dfrac{{2{W^2}L + {W^2}L}}{{8\pi {R^2}Y}}$
$ \Rightarrow E = \dfrac{{3{W^2}L}}{{8\pi {R^2}Y}}$.
The potential energy of the wire is equal to $E = \dfrac{{3{W^2}L}}{{8\pi {R^2}Y}}$. The correct option for this problem is option B.
Note: The students are advised to understand and remember the formula of the potential energy of the wire as it is very useful in solving these kinds of problems. The change of the length happens due to the applied force or the load due to weight.
Formula used: The formula of the potential energy is given by,
$ \Rightarrow E = \dfrac{{{F^2}L}}{{2AY}}$
Where force is F, the original length is L, the area is A and Young’s modulus is Y.
Complete step by step solution:
It is given in the problem that the two wires of the same material (young’s modulus Y) and same length L but radii R and 2R respectively are joined end to end and a weight W is suspended from the combination and we need to find the elastic potential energy in the system in equilibrium condition.
The formula of the potential energy is given by,
$ \Rightarrow E = \dfrac{{{F^2}L}}{{2AY}}$
Where force is F, the original length is L, the area is A and Young’s modulus is Y.
The potential energy of the wire is equal to,
$ \Rightarrow E = \dfrac{{{F^2}L}}{{2AY}}$
The elongation is taking place in the two parts of the wires.
The potential energy of the system is equal to,
$ \Rightarrow E = \dfrac{{{W^2}L}}{{2{A_1}Y}} + \dfrac{{{W^2}L}}{{2{A_2}Y}}$
$ \Rightarrow E = \dfrac{{{W^2}L}}{{2\left( {\pi {R^2}} \right)Y}} + \dfrac{{{W^2}L}}{{2\pi {{\left( {2R} \right)}^2}Y}}$
$ \Rightarrow E = \dfrac{{{W^2}L}}{{2\pi {R^2}Y}} + \dfrac{{{W^2}L}}{{8\pi {R^2}Y}}$
$ \Rightarrow E = \dfrac{{2{W^2}L + {W^2}L}}{{8\pi {R^2}Y}}$
$ \Rightarrow E = \dfrac{{3{W^2}L}}{{8\pi {R^2}Y}}$.
The potential energy of the wire is equal to $E = \dfrac{{3{W^2}L}}{{8\pi {R^2}Y}}$. The correct option for this problem is option B.
Note: The students are advised to understand and remember the formula of the potential energy of the wire as it is very useful in solving these kinds of problems. The change of the length happens due to the applied force or the load due to weight.
Recently Updated Pages
JEE Main 2023 (January 29th Shift 1) Physics Question Paper with Answer Key

JEE Main 2021 July 20 Shift 2 Question Paper with Answer Key

JEE Main 2023 January 30 Shift 2 Question Paper with Answer Key

JEE Main 2022 (June 24th Shift 1) Physics Question Paper with Answer Key

Equivalent Capacitance - Important Concepts and Tips for JEE

JEE Main 2023 (April 6th Shift 1) Physics Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

Degree of Dissociation and Its Formula With Solved Example for JEE

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

Motion in a Straight Line Class 11 Notes: CBSE Physics Chapter 2

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry




