
What do you understand by ‘Sharpness of resonance’ for a series LCR resonant circuit? How is it related to the quality factor ‘Q’ of the circuit? Using the graphs given in the diagram, explain the factors which affect it. For which graph is the resistance (R) minimum? KR 0 = 0, 0

Answer
460.8k+ views
Hint : In this question, we find’ what is ‘sharpness of resonance’ for a
Complete step by step answer:
(A) We know that in
Here,
At Resonance in
We can write
On further solving we can write the equation as,
We take square root on both sides of the equation,
This equation is known as the resonance frequency.
Further, impedance is minimum at resonance.
This means that the current becomes maximum,
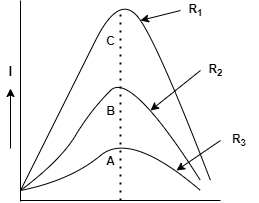
B) The figure shows the variation of current amplitude
C) Quality factor or
The
Note: To understand this question we have to study
Complete step by step answer:
(A) We know that in
Here,
At Resonance in
We can write
On further solving we can write the equation as,
We take square root on both sides of the equation,
This equation is known as the resonance frequency.
Further, impedance is minimum at resonance.
This means that the current becomes maximum,
B) The figure shows the variation of current amplitude
C) Quality factor or
The
Note: To understand this question we have to study
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




