
Volume of the tetrahedron whose vertices are represented by the position vectors,
A.
B.
C.
D.None
Answer
476.7k+ views
Hint: We are asked to find the volume of the tetrahedron. For this, recall the formula for volume of a tetrahedron. The vertices of the tetrahedron are given, draw a tetrahedron showing the vertices and find the edges. Using these edges find the volume of the tetrahedron
Complete step-by-step answer:
Given, the vertices of the tetrahedron are
Let us draw a tetrahedron with the given vertices,
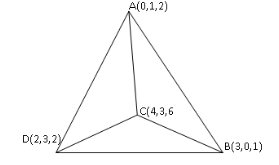
In the form of vectors, the points can be written as,
Volume of a tetrahedron can be expressed as,
where
Now, let us find the edges of the given tetrahedron
Vectors
Let us solve this part by part.
First let us take
Putting the values of
Now, putting the value of
Therefore, the volume of the tetrahedron is
So, the correct answer is “Option C”.
Note: There are few more important geometrical shapes in three-dimension, these are cube, cuboid, pentagonal prism, hexagonal prism, octahedron, cone, cylinder, ellipsoid. The number of vertices, faces and edges are different for different shapes. Students usually get confused between the number of vertices and edges of different shapes which leads to an incorrect answer so, always try to remember the number of vertices, edges and faces for each shape.
Complete step-by-step answer:
Given, the vertices of the tetrahedron are
Let us draw a tetrahedron with the given vertices,

In the form of vectors, the points can be written as,
Volume of a tetrahedron can be expressed as,
where
Now, let us find the edges of the given tetrahedron
Vectors
Let us solve this part by part.
First let us take
Putting the values of
Now, putting the value of
Therefore, the volume of the tetrahedron is
So, the correct answer is “Option C”.
Note: There are few more important geometrical shapes in three-dimension, these are cube, cuboid, pentagonal prism, hexagonal prism, octahedron, cone, cylinder, ellipsoid. The number of vertices, faces and edges are different for different shapes. Students usually get confused between the number of vertices and edges of different shapes which leads to an incorrect answer so, always try to remember the number of vertices, edges and faces for each shape.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




