Question

How is wavelength determined for a longitudinal wave?

Answer 1

Hint: Recall that a longitudinal wave is composed of progressions of successive high-pressure and low-pressure regions called compressions and rarefactions respectively. We also know that the wavelength for any wave in general is defined as the distance between any two identical points of phase and amplitude. In such a case, determine the probable identical points on a longitudinal wave following which you should be able to deduce how the wavelength of such a wave can be determined.

Complete Solution:
Let us begin by understanding what a longitudinal wave is and the characteristic properties of the same, following which we shall define the wavelength of a wave.

Longitudinal waves are mechanical waves composed of compressions and rarefactions that propagate through a medium in the same direction as the wave motion. They are thus called compression waves, and are sometimes called pressure waves when they produce variations in pressure that propagate through the material of the medium. Sound waves are an example of longitudinal waves. A good visualization of the propagation of a longitudinal wave can be given by the behaviour of a Slinky toy when stretched and released, where the distance between its coils increases and decreases as it moves.

Transverse waves, on the other hand, are composed of crests and troughs that propagate through a medium in a direction perpendicular to the wave motion. Thus, a crest is analogous to a region of high pressure (compression) on a longitudinal wave and a trough is analogous to a region of low pressure (rarefaction) on a longitudinal wave.

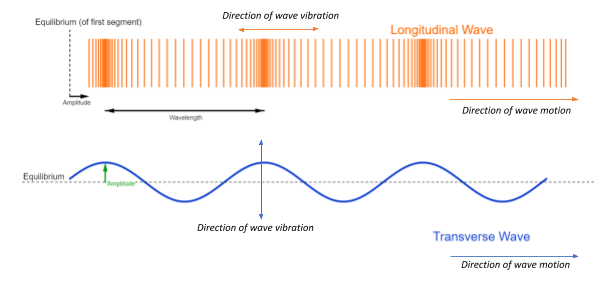
The wavelength of a transverse wave is defined as the distance between two successive crests or troughs of the wave measures along the direction of motion of the wave.

From our previous analogy, we can thus conclude that the wavelength of a longitudinal wave is the distance between successive compressions (high pressure regions) or successive rarefactions (regions of low pressure), as shown in the figure where the wavelength is defined over two successive compressions for the longitudinal wave and over two successive crests for the transverse wave.

Though the wavelength is easier to define over compressions and rarefactions since they are visually prominent, we can also obtain the wavelength by taking the distance between any two identical points (of amplitude and phase) on a wave.

Note:
In addition to our discussion above, it is also important to understand the following distinctions between longitudinal and transverse waves.
In longitudinal waves, the particles of the medium move parallel to the propagation of the wave, thus acting in one dimension, whereas in transverse waves, the particles of the medium move perpendicular to the direction of propagation of the wave, thus acting in two dimensions.
Generally, transverse waves do not require a material medium for their propagation whereas longitudinal waves cannot propagate without a material medium, which is a consequence of why light travels through space/vacuum but sound waves do not.